Full solution
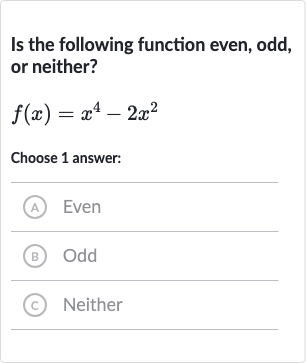
Q. Is the following function even, odd, or neither?Choose answer:(A) Even(B) Odd(C) Neither
- Define function and determine f(-x): Define the function f(x) and determine f(-x).The function given is . To determine if the function is even, odd, or neither, we need to evaluate and compare it to .
- Substitute in : Substitute for in to find .
- Simplify : Simplify the right side of the function. simplifies to because and .
- Compare and : Compare and .We have and . Since , the function is an even function.