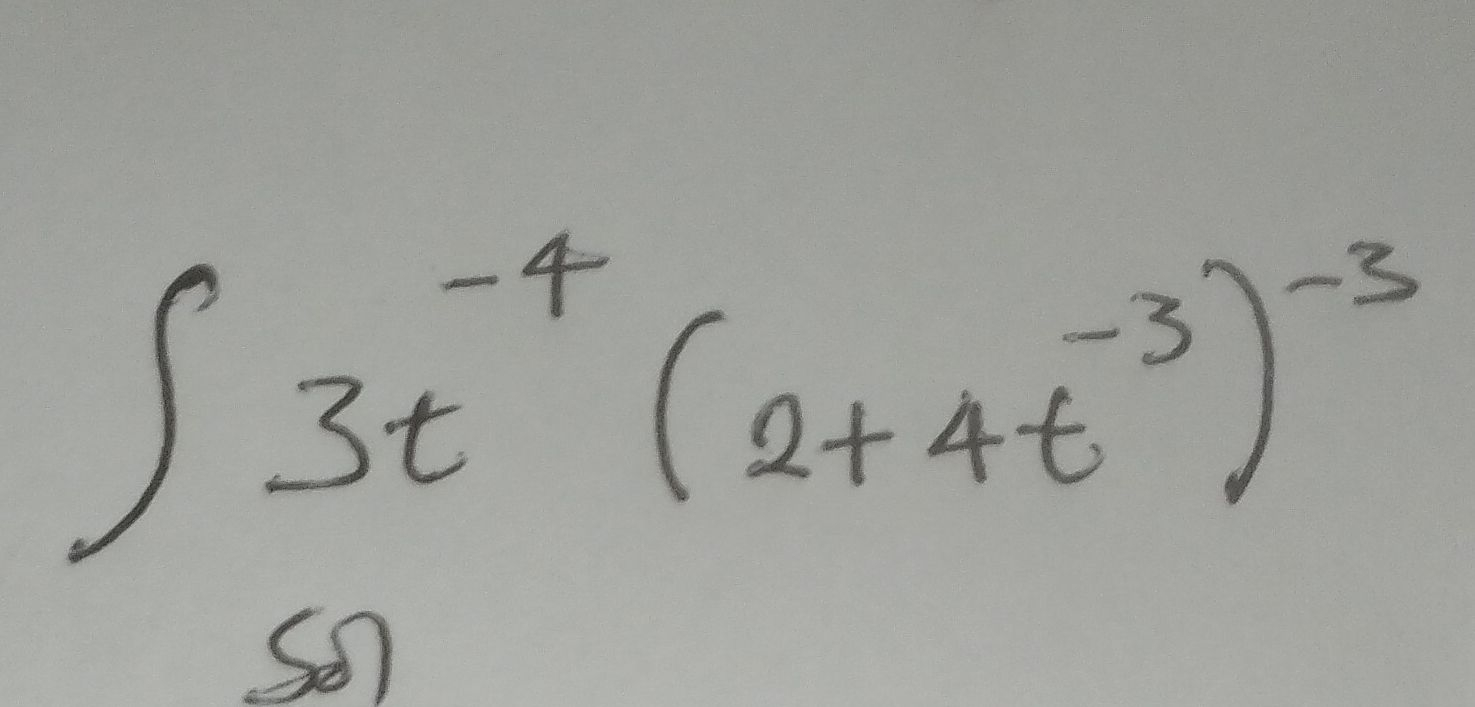AI tutor
Full solution
Q. Integrate.
- Identify Substitution: Given the integral to solve:Identify a substitution that can simplify the integral.Let . Then, differentiate with respect to to find .
- Find du: Solve for in terms of and .Now, substitute and into the integral.
- Substitute u and dt: Integrate with respect to .
- Integrate with u: Substitute back the original expression for in terms of .