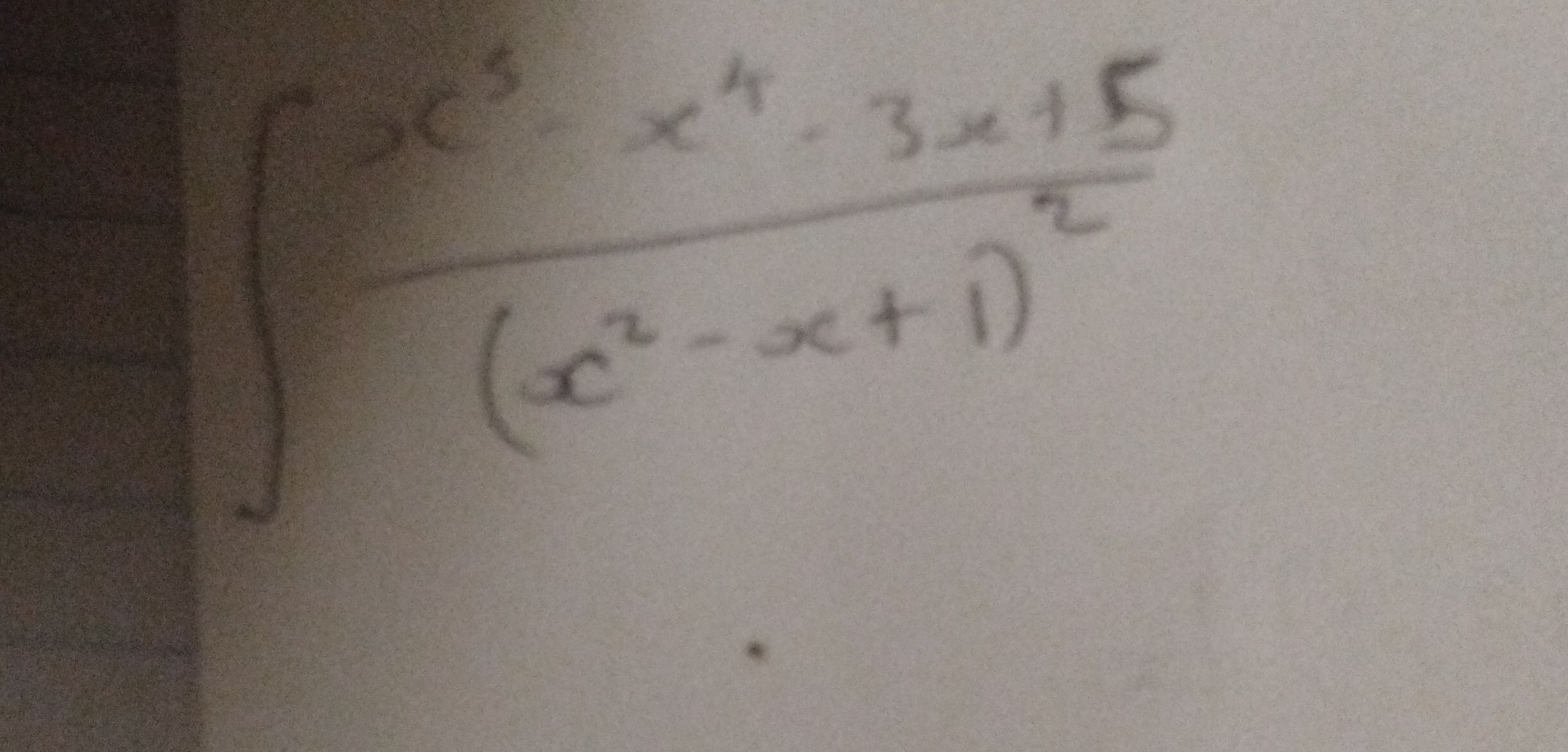Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Evaluate the integral: int(x^(5)-x^(4)-3x+5)/((x^(2)-x+1)^(2))

Evaluate the integral:∫x5−x4−3x+5(x2−x+1)2 \int \frac{x^{5}-x^{4}-3 x+5}{\left(x^{2}-x+1\right)^{2}}

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Calculusright chevron icon
Find indefinite integrals using the substitutionright chevron icon

Full solution

Q. Evaluate the integral:∫x5−x4−3x+5(x2−x+1)2 \int \frac{x^{5}-x^{4}-3 x+5}{\left(x^{2}-x+1\right)^{2}}
  1. Perform Long Division: We are given the integral:\newline∫(x5−x4−3x+5(x2−x+1)2)dx\int\left(\frac{x^5 - x^4 - 3x + 5}{(x^2 - x + 1)^2}\right)dx\newlineTo solve this integral, we will use the method of partial fractions. However, since the degree of the numerator is higher than the degree of the denominator, we first need to perform polynomial long division.
  2. Rewrite Integral: Perform polynomial long division of (x5−x4−3x+5)(x^5 - x^4 - 3x + 5) by (x2−x+1)(x^2 - x + 1).
  3. Integrate Polynomials: After performing the long division, we get: x3+x2+2x+x^3 + x^2 + 2x + (remainder) where the remainder is a polynomial of degree less than 22.
  4. Find Remainder: Now we rewrite the integral as:\newline∫(x3+x2+2x) dx+∫(remainder(x2−x+1)2) dx\int(x^3 + x^2 + 2x)\,dx + \int(\frac{\text{remainder}}{(x^2 - x + 1)^2})\,dx\newlineThe first integral can be solved directly, while the second integral will require partial fractions.
  5. Determine AA and BB: Integrate x3x^3, x2x^2, and 2x2x separately:\newline∫(x3) dx=14x4\int(x^3)\,dx = \frac{1}{4}x^4\newline∫(x2) dx=13x3\int(x^2)\,dx = \frac{1}{3}x^3\newline∫(2x) dx=x2\int(2x)\,dx = x^2
  6. Partial Fraction Decomposition: Now we need to find the remainder from the long division to proceed with the partial fraction decomposition.
  7. Solve for CC, DD, EE, FF: After finding the remainder, we have:\newlineremainder = (Ax+B)(Ax + B)\newlineWe need to determine AA and BB by equating coefficients from the long division.
  8. Integrate Terms: After determining AA and BB, we can write the remainder as: remainder=(Ax+B)\text{remainder} = (Ax + B) Now we can proceed with the partial fraction decomposition for the integral of the remainder over (x2−x+1)2(x^2 - x + 1)^2.
  9. Combine Final Answer: The partial fraction decomposition for the remainder will be in the form of:\newline(Ax+B)/((x2−x+1)2)=(Cx+D)/(x2−x+1)+(Ex+F)/(x2−x+1)2(Ax + B) / ((x^2 - x + 1)^2) = (Cx + D) / (x^2 - x + 1) + (Ex + F) / (x^2 - x + 1)^2\newlineWe need to solve for CC, DD, EE, and FF.
  10. Combine Final Answer: The partial fraction decomposition for the remainder will be in the form of:\newline(Ax+B)/((x2−x+1)2)=(Cx+D)/(x2−x+1)+(Ex+F)/(x2−x+1)2(Ax + B) / ((x^2 - x + 1)^2) = (Cx + D) / (x^2 - x + 1) + (Ex + F) / (x^2 - x + 1)^2\newlineWe need to solve for CC, DD, EE, and FF.After solving for CC, DD, EE, and FF, we can integrate each term separately:\newline∫((Cx+D)/(x2−x+1)) dx+∫((Ex+F)/(x2−x+1)2) dx\int((Cx + D) / (x^2 - x + 1))\,dx + \int((Ex + F) / (x^2 - x + 1)^2)\,dx
  11. Combine Final Answer: The partial fraction decomposition for the remainder will be in the form of:\newline(Ax+B)/((x2−x+1)2)=(Cx+D)/(x2−x+1)+(Ex+F)/(x2−x+1)2(Ax + B) / ((x^2 - x + 1)^2) = (Cx + D) / (x^2 - x + 1) + (Ex + F) / (x^2 - x + 1)^2\newlineWe need to solve for CC, DD, EE, and FF.After solving for CC, DD, EE, and FF, we can integrate each term separately:\newline∫((Cx+D)/(x2−x+1)) dx+∫((Ex+F)/(x2−x+1)2) dx\int((Cx + D) / (x^2 - x + 1))\,dx + \int((Ex + F) / (x^2 - x + 1)^2)\,dxThe integration of these terms may involve completing the square and using substitution to solve the integrals.
  12. Combine Final Answer: The partial fraction decomposition for the remainder will be in the form of:\newline(Ax+B)/((x2−x+1)2)=(Cx+D)/(x2−x+1)+(Ex+F)/(x2−x+1)2(Ax + B) / ((x^2 - x + 1)^2) = (Cx + D) / (x^2 - x + 1) + (Ex + F) / (x^2 - x + 1)^2\newlineWe need to solve for CC, DD, EE, and FF.After solving for CC, DD, EE, and FF, we can integrate each term separately:\newline∫((Cx+D)/(x2−x+1)) dx+∫((Ex+F)/(x2−x+1)2) dx\int((Cx + D) / (x^2 - x + 1))\,dx + \int((Ex + F) / (x^2 - x + 1)^2)\,dxThe integration of these terms may involve completing the square and using substitution to solve the integrals.After integrating, we combine all the terms to get the final answer.

More problems from Find indefinite integrals using the substitution

Question
Evaluate the integral.\newline∫−x4−3xdx \int-x 4^{-3 x} d x \newlineAnswer:
Get tutor helpright-arrow

Posted 9 months ago

Question
Evaluate the integral.\newline∫6x3e−2xdx \int 6 x^{3} e^{-2 x} d x \newlineAnswer:
Get tutor helpright-arrow

Posted 9 months ago

Question
Evaluate the integral.\newline∫x53xdx \int x 5^{3 x} d x \newlineAnswer:
Get tutor helpright-arrow

Posted 9 months ago

Question
Evaluate the integral.\newline∫−2xe2xdx \int-2 x e^{2 x} d x \newlineAnswer:
Get tutor helpright-arrow

Posted 9 months ago

Question
Evaluate the integral.\newline∫−2xcos⁡(−2x)dx \int-2 x \cos (-2 x) d x \newlineAnswer:
Get tutor helpright-arrow

Posted 9 months ago

Question
Evaluate the integral.\newline∫−xcos⁡(−3x)dx \int-x \cos (-3 x) d x \newlineAnswer:
Get tutor helpright-arrow

Posted 9 months ago

Question
Evaluate the integral.\newline∫6x253xdx \int 6 x^{2} 5^{3 x} d x \newlineAnswer:
Get tutor helpright-arrow

Posted 9 months ago

Question
Evaluate the integral.\newline∫−6x4−4xdx \int-6 x 4^{-4 x} d x \newlineAnswer:
Get tutor helpright-arrow

Posted 9 months ago

Question
Evaluate the integral.\newline∫6x223xdx \int 6 x^{2} 2^{3 x} d x \newlineAnswer:
Get tutor helpright-arrow

Posted 9 months ago

Question
Evaluate the integral.\newline∫−3xsin⁡(−2x)dx \int-3 x \sin (-2 x) d x \newlineAnswer:
Get tutor helpright-arrow

Posted 9 months ago

View all (263)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant