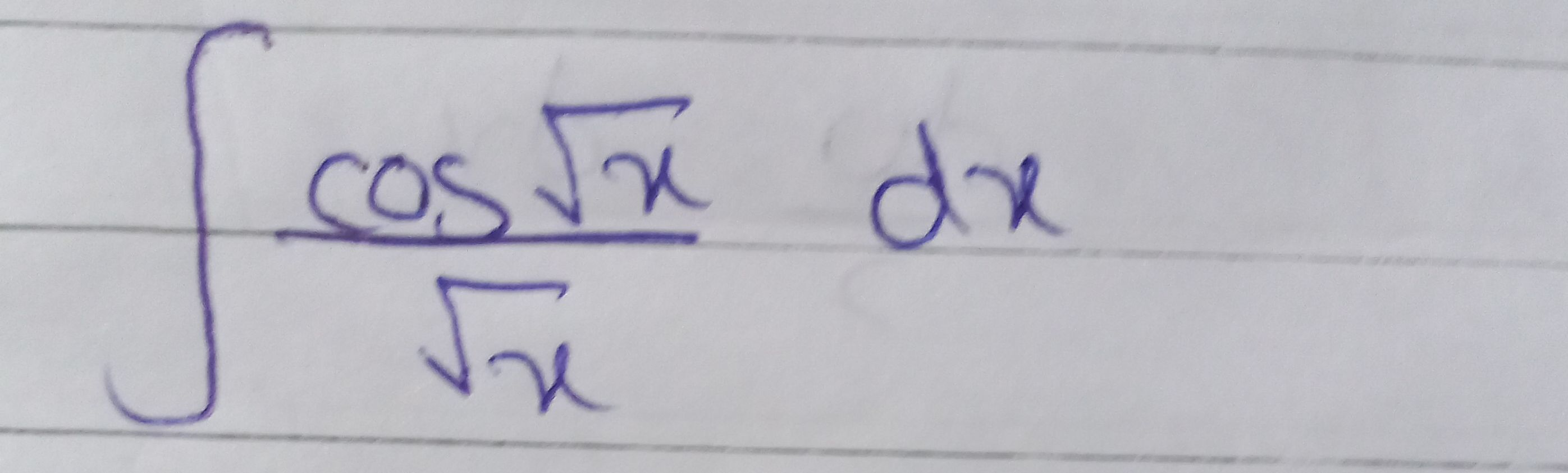Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

int(cos sqrtx)/(sqrtx)dx=

∫cos⁡xxdx \int \frac{\cos \sqrt{x}}{\sqrt{x}} d x =

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Calculusright chevron icon
Find indefinite integrals using the substitution and by partsright chevron icon

Full solution

Q. ∫cos⁡xxdx \int \frac{\cos \sqrt{x}}{\sqrt{x}} d x =
  1. Substitution step: Let u=xu = \sqrt{x}, which implies that x=u2x = u^2 and dx=2u dudx = 2u \, du. This substitution will simplify the integral.
  2. Rewrite in terms of uu: Rewrite the integral in terms of uu. The integral becomes ∫cos⁡(u)u⋅2u du\int\frac{\cos(u)}{u} \cdot 2u \, du, where we have replaced x\sqrt{x} with uu and dxdx with 2u du2u \, du.
  3. Simplify the integral: Simplify the integral by canceling the uu in the denominator with one of the uu's in the numerator. The integral now is 2×∫(cos⁡(u)) du2 \times \int(\cos(u)) \, du.
  4. Integrate cos⁡(u)\cos(u): Integrate 2×∫(cos⁡(u)) du2 \times \int(\cos(u)) \, du with respect to uu. The integral of cos⁡(u)\cos(u) with respect to uu is sin⁡(u)\sin(u).
  5. Multiply by 22: Multiply the result of the integration by 22. The result is 2sin⁡(u)+C2\sin(u) + C, where CC is the constant of integration.
  6. Substitute back xx: Substitute back the original variable xx into the result. Since u=xu = \sqrt{x}, the result is 2sin⁡(x)+C2\sin(\sqrt{x}) + C.

More problems from Find indefinite integrals using the substitution and by parts

Question
Evaluate the integral.\newline∫−x4−3xdx \int-x 4^{-3 x} d x \newlineAnswer:
Get tutor helpright-arrow

Posted 3 months ago

Question
Evaluate the integral.\newline∫6x3e−2xdx \int 6 x^{3} e^{-2 x} d x \newlineAnswer:
Get tutor helpright-arrow

Posted 3 months ago

Question
Evaluate the integral.\newline∫x53xdx \int x 5^{3 x} d x \newlineAnswer:
Get tutor helpright-arrow

Posted 3 months ago

Question
Evaluate the integral.\newline∫−2xe2xdx \int-2 x e^{2 x} d x \newlineAnswer:
Get tutor helpright-arrow

Posted 3 months ago

Question
Evaluate the integral.\newline∫−2xcos⁡(−2x)dx \int-2 x \cos (-2 x) d x \newlineAnswer:
Get tutor helpright-arrow

Posted 3 months ago

Question
Evaluate the integral.\newline∫−xcos⁡(−3x)dx \int-x \cos (-3 x) d x \newlineAnswer:
Get tutor helpright-arrow

Posted 3 months ago

Question
Evaluate the integral.\newline∫6x253xdx \int 6 x^{2} 5^{3 x} d x \newlineAnswer:
Get tutor helpright-arrow

Posted 3 months ago

Question
Evaluate the integral.\newline∫−6x4−4xdx \int-6 x 4^{-4 x} d x \newlineAnswer:
Get tutor helpright-arrow

Posted 3 months ago

Question
Evaluate the integral.\newline∫6x223xdx \int 6 x^{2} 2^{3 x} d x \newlineAnswer:
Get tutor helpright-arrow

Posted 3 months ago

Question
Evaluate the integral.\newline∫−3xsin⁡(−2x)dx \int-3 x \sin (-2 x) d x \newlineAnswer:
Get tutor helpright-arrow

Posted 3 months ago

View all (147)