Given the following point on the unit circle, find the angle, to the nearest tenth of a degree (if necessary), of the terminal side through that point, 0^(@)

Question

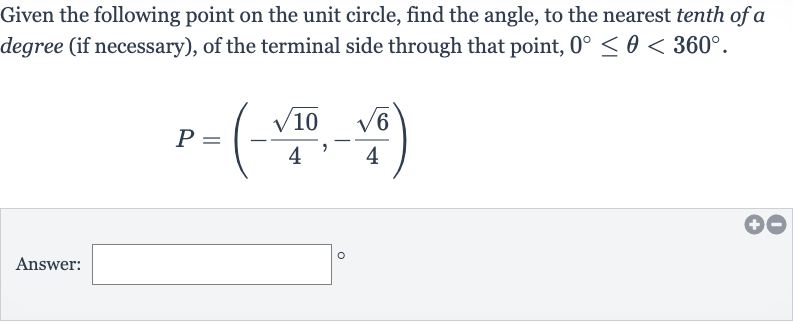
Given the following point on the unit circle, find the angle, to the nearest tenth of a degree (if necessary), of the terminal side through that point,  0^(@) <= theta < 360^(@).  P=(-(sqrt10)/(4),-(sqrt6)/(4)) Answer:

Bytelearn · Accepted Answer

Point Location: The point P=(-(sqrt10)/(4),-(sqrt6)/(4)) lies in the third quadrant of the unit circle because both x and y coordinates are negative.Angle Calculation: To find the angle theta, we can use the arctangent function. However, since we are in the third quadrant, we need to add 180° to the result of the arctangent of the y-coordinate over the x-coordinate to get the angle in the correct quadrant.Arctangent Calculation: Calculate the arctangent of the y-coordinate over the x-coordinate. Note that since both x and y are negative, the ratio will be positive: arctan(y/x) = arctan((-(sqrt6)/(4))/(-(sqrt10)/(4))) = arctan(sqrt6/sqrt10) = arctan(sqrt(6/10)) = arctan(sqrt(3/5)).Calculate Angle: Use a calculator to find the arctan(sqrt(3/5)) and then add 180° to find the angle in the third quadrant: theta = arctan(sqrt(3/5)) + 180°.Final Angle Calculation: After calculating, we find that arctan(sqrt(3/5)) is approximately 36.87°. Adding 180° gives us: theta = 36.87° + 180° = 216.87°.Round Angle: Round the angle to the nearest tenth of a degree: theta ≈ 216.9°.

Given the following point on the unit circle, find the angle, to the nearest tenth of a degree (if necessary), of the terminal side through that point, 0^{\circ} \leq \theta<360^{\circ} . $\newline$ $P=\left(-\frac{\sqrt{10}}{4},-\frac{\sqrt{6}}{4}\right)$ $\newline$ Answer:

Full solution

More problems from Find indefinite integrals using the substitution

Related topics