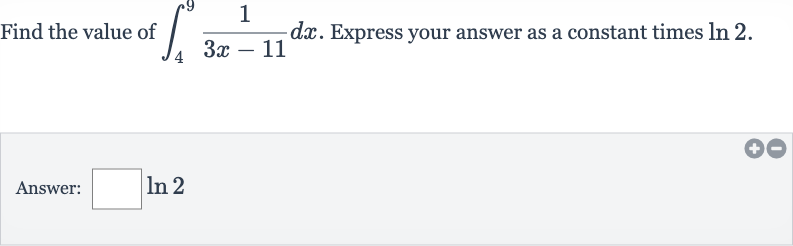
Find the value of int_(4)^(9)(1)/(3x-11)dx. Express your answer as a constant times ln 2. Answer: ◻ln 2

Question

Find the value of  int_(4)^(9)(1)/(3x-11)dx. Express your answer as a constant times  ln 2. Answer:  ◻ln 2

Bytelearn · Accepted Answer

Recognize standard form: Recognize the integral as a standard form. The integral ∫(1)/(3x-11)dx is a standard form of the integral of a function 1/(ax+b). The antiderivative of 1/(ax+b) is (1/a)ln|ax+b| + C, where C is the constant of integration.Calculate antiderivative: Calculate the antiderivative. To find the antiderivative of (1)/(3x-11), we can use the formula mentioned above with a = 3 and b = -11. Thus, the antiderivative is (1/3)ln|3x-11| + C.Evaluate definite integral: Evaluate the definite integral from 4 to 9. We need to evaluate the antiderivative at the upper and lower limits of the integral and subtract the lower evaluation from the upper evaluation. ∫_(4)^(9)(1)/(3x-11)dx = [(1/3)ln|3x-11|] from x=4 to x=9 = (1/3)ln|3(9)-11| - (1/3)ln|3(4)-11| = (1/3)ln|27-11| - (1/3)ln|12-11| = (1/3)ln|16| - (1/3)ln|1|Simplify expression: Simplify the expression. Since ln|1| = 0, we can simplify the expression to: (1/3)ln|16| - (1/3)(0) = (1/3)ln(16) = (1/3)ln(2^4) = (1/3)(4)ln(2) = (4/3)ln(2)Express final answer: Express the answer as a constant times ln 2. The final answer is (4/3)ln(2), which is already expressed as a constant times ln 2.

Find the value of $\int_{4}^{9} \frac{1}{3 x-11} d x$ . Express your answer as a constant times $\ln 2$ . $\newline$ Answer: $\square \ln 2$

Full solution

More problems from Find indefinite integrals using the substitution and by parts

Related topics

Find the value of ∫4913x−11dx \int_{4}^{9} \frac{1}{3 x-11} d x ∫49​3x−111​dx. Express your answer as a constant times ln⁡2 \ln 2 ln2.\newlineAnswer: □ln⁡2 \square \ln 2 □ln2

Find the value of $\int_{4}^{9} \frac{1}{3 x-11} d x$ . Express your answer as a constant times $\ln 2$ . $\newline$ Answer: $\square \ln 2$