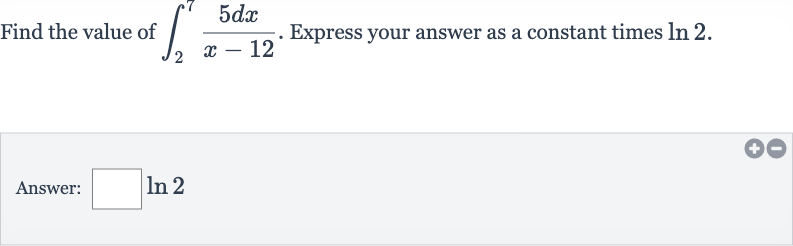
Find the value of int_(2)^(7)(5dx)/(x-12). Express your answer as a constant times ln 2. Answer: ◻ln 2

Question

Find the value of  int_(2)^(7)(5dx)/(x-12). Express your answer as a constant times  ln 2. Answer:  ◻ln 2

Bytelearn · Accepted Answer

Recognize standard form: Recognize the integral as a standard form. The integral $\int \frac{5dx}{x-12}$ can be recognized as a standard form of the integral $\int \frac{1}{u}du$, where $u = x - 12$.Perform substitution: Perform a substitution. Let $u = x - 12$, then $du = dx$. The integral becomes $\int \frac{5du}{u}$.Integrate using logarithm: Integrate using the natural logarithm. The integral of $\frac{1}{u}$ with respect to $u$ is $\ln|u|$. Therefore, the integral becomes $5\ln|u| + C$, where $C$ is the constant of integration.Substitute back for u: Substitute back for $u$. Replace $u$ with $x - 12$ to get $5\ln|x - 12| + C$.Evaluate definite integral: Evaluate the definite integral from 2 to 7. We need to evaluate $5\ln|x - 12|$ from $x = 2$ to $x = 7$. This gives us $5\ln|7 - 12| - 5\ln|2 - 12|$.Simplify expression: Simplify the expression. Since $7 - 12 = -5$ and $2 - 12 = -10$, and the absolute value of a negative number is positive, we have $5\ln(5) - 5\ln(10)$.Use logarithm properties: Use the properties of logarithms. We can use the property $\ln(a) - \ln(b) = \ln(\frac{a}{b})$ to combine the logarithms: $5\ln(\frac{5}{10})$.Simplify fraction in logarithm: Simplify the fraction inside the logarithm. The fraction $\frac{5}{10}$ simplifies to $\frac{1}{2}$, so the expression becomes $5\ln(\frac{1}{2})$.Bring constant outside logarithm: Use the property of logarithms to bring the constant outside the logarithm. Since $\ln(a^b) = b\ln(a)$, we can write $5\ln(\frac{1}{2})$ as $-5\ln(2)$ because $\ln(\frac{1}{2}) = \ln(2^{-1}) = -\ln(2)$.Express final answer: Express the final answer. The value of the definite integral from 2 to 7 of $\frac{5dx}{x-12}$ is $-5\ln(2)$.

Find the value of $\int_{2}^{7} \frac{5 d x}{x-12}$ . Express your answer as a constant times $\ln 2$ . $\newline$ Answer: $\square \ln 2$

Full solution

More problems from Find indefinite integrals using the substitution and by parts

Related topics

Find the value of ∫275dxx−12 \int_{2}^{7} \frac{5 d x}{x-12} ∫27​x−125dx​. Express your answer as a constant times ln⁡2 \ln 2 ln2.\newlineAnswer: □ln⁡2 \square \ln 2 □ln2

Find the value of $\int_{2}^{7} \frac{5 d x}{x-12}$ . Express your answer as a constant times $\ln 2$ . $\newline$ Answer: $\square \ln 2$