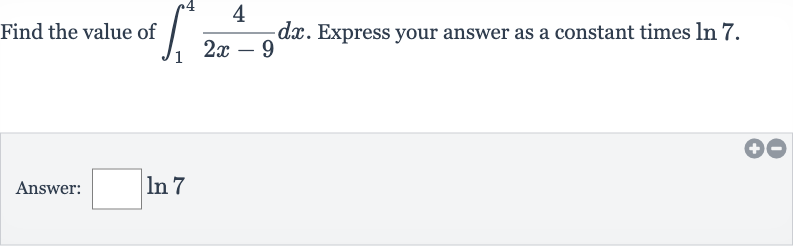
Find the value of int_(1)^(4)(4)/(2x-9)dx. Express your answer as a constant times ln 7. Answer: ◻ln 7

Question

Find the value of  int_(1)^(4)(4)/(2x-9)dx. Express your answer as a constant times  ln 7. Answer:  ◻ln 7

Bytelearn · Accepted Answer

Recognize standard form: Recognize the integral as a standard form. The integral ∫(4)/(2x-9)dx can be recognized as a standard form of the integral of a function of the form 1/u, which is ln|u| + C, where C is the constant of integration.Perform substitution to simplify: Perform a substitution to simplify the integral. Let u = 2x - 9, then du/dx = 2, or dx = du/2. The integral becomes ∫(4)/(u) * (du/2).Simplify the integral: Simplify the integral. The integral simplifies to 2∫(1/u)du, which is 2ln|u| + C.Substitute back for u: Substitute back for u. Substitute back u = 2x - 9 into the integral to get 2ln|2x - 9| + C.Evaluate definite integral: Evaluate the definite integral from 1 to 4. We need to evaluate 2ln|2x - 9| from x = 1 to x = 4. This gives us 2ln|2(4) - 9| - 2ln|2(1) - 9|, which simplifies to 2ln|8 - 9| - 2ln|2 - 9|.Simplify expression: Simplify the expression. Since ln|8 - 9| = ln|1| = 0 and ln|2 - 9| = ln|-7| = ln|7| (because the natural logarithm function is even), the expression simplifies to 0 - 2ln|7|.Final answer: Simplify the final answer. The final answer is -2ln|7|, which can be expressed as -2ln7 because 7 is positive and we do not need the absolute value.

Find the value of $\int_{1}^{4} \frac{4}{2 x-9} d x$ . Express your answer as a constant times $\ln 7$ . $\newline$ Answer: $\square \ln 7$

Full solution

More problems from Find indefinite integrals using the substitution and by parts

Related topics

Find the value of ∫1442x−9dx \int_{1}^{4} \frac{4}{2 x-9} d x ∫14​2x−94​dx. Express your answer as a constant times ln⁡7 \ln 7 ln7.\newlineAnswer: □ln⁡7 \square \ln 7 □ln7

Find the value of $\int_{1}^{4} \frac{4}{2 x-9} d x$ . Express your answer as a constant times $\ln 7$ . $\newline$ Answer: $\square \ln 7$