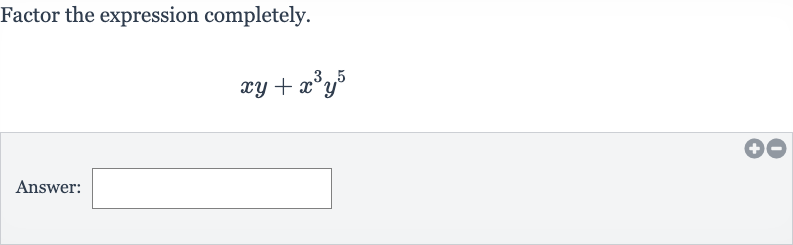AI tutor
Full solution
Q. Factor the expression completely.Answer:
- Identify Common Factors: Identify the common factors in both terms of the expression and . Both terms have an in common.
- Factor Out Common Factor: Factor out the common factor from both terms.
- Check for Further Factoring: Check if the remaining expression inside the parentheses can be factored further. cannot be factored further over the real numbers because it is not a difference of squares and does not have any common factors.
- Write Final Factored Form: Write down the final factored form of the expression.The completely factored form of the expression is .