AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
Evaluate the integral.Answer:
Full solution
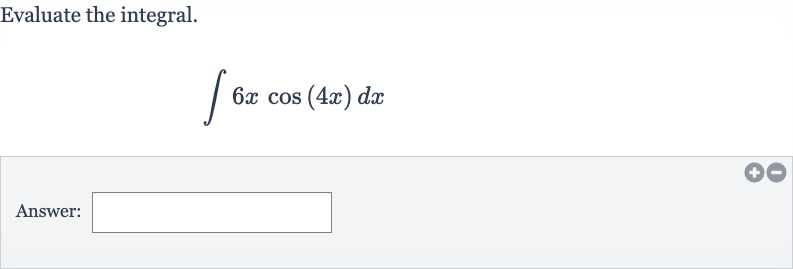
Q. Evaluate the integral.Answer:
- Identify integral: Identify the integral to be solved.We need to evaluate the integral of the function with respect to , which is written as .
- Use integration by parts: Use integration by parts.Integration by parts is given by the formula , where and are parts of the integrand. We choose and as follows:Let , which implies .Let , which implies after integrating with respect to .
- Apply integration by parts: Apply the integration by parts formula.Using the chosen and , we have:
- Integrate remaining integral: Integrate the remaining integral.Now we need to integrate . The antiderivative of with respect to is . So we have:\frac{3}{2}\int \sin(4x) \, dx = \frac{3}{2}\left(-\frac{1}{4}\cos(4x)\right) + C\(\newline= -\frac{3}{8}\cos(4x) + C\), where is the constant of integration.
- Combine final answer: Combine the results to get the final answer.Putting it all together, we have:
