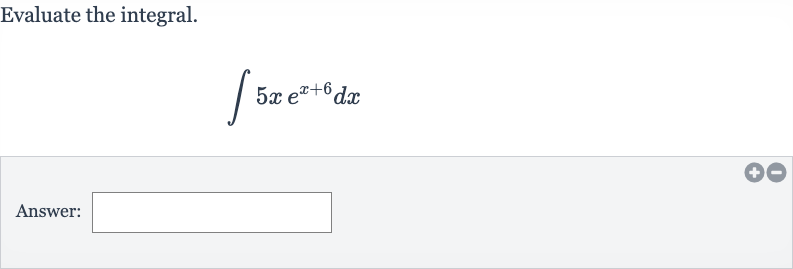Evaluate the integral. int5xe^(x+6)dx Answer:

Question

Evaluate the integral.  int5xe^(x+6)dx Answer:

Bytelearn · Accepted Answer

Set Up Integral: Let's start by setting up the integral that we need to evaluate: I = ∫5xe^(x+6)dx We can see that this is an integration problem that requires the use of integration by parts, which is given by the formula ∫u dv = uv - ∫v du, where u and dv are parts of the integrand that we choose.Choose u and dv: Choose u and dv for the integration by parts. A good choice here is to let u = 5x, because its derivative will be simpler, and dv = e^(x+6)dx, because its integral is straightforward. So we have: u = 5x, which means du = 5dx dv = e^(x+6)dx, which means v = e^(x+6) since the derivative of (x+6) is 1.Apply Integration by Parts: Apply the integration by parts formula: I = uv - ∫v du Substitute u, du, v, and dv into the formula: I = 5x * e^(x+6) - ∫e^(x+6) * 5dxIntegrate Second Term: Now we integrate the second term: ∫e^(x+6) * 5dx = 5 * e^(x+6) So the integral becomes: I = 5x * e^(x+6) - 5 * e^(x+6)Simplify Expression: Simplify the expression by factoring out e^(x+6): I = e^(x+6) * (5x - 5)Add Constant of Integration: Finally, we can add the constant of integration, C, to our result: I = e^(x+6) * (5x - 5) + C This is the indefinite integral of the given function.

Evaluate the integral. $\newline$ $\int 5 x e^{x+6} d x$ $\newline$ Answer:

Full solution

More problems from Find indefinite integrals using the substitution and by parts

Related topics

Evaluate the integral.\newline∫5xex+6dx \int 5 x e^{x+6} d x ∫5xex+6dx\newlineAnswer:

Evaluate the integral. $\newline$ $\int 5 x e^{x+6} d x$ $\newline$ Answer: