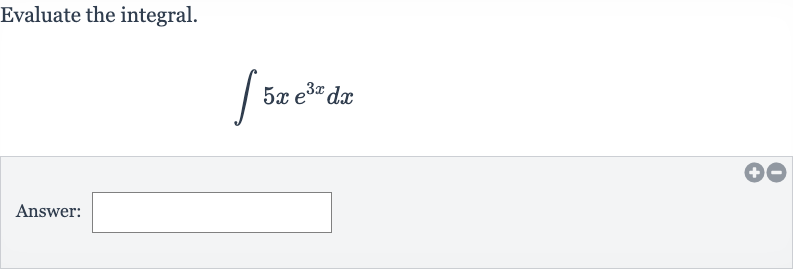
Evaluate the integral. int5xe^(3x)dx Answer:

Question

Evaluate the integral.  int5xe^(3x)dx Answer:

Bytelearn · Accepted Answer

Identify integral: Identify the integral to be solved. We need to evaluate the integral of the function 5xe^(3x) with respect to x. This is an integration problem that requires the use of integration by parts, which is based on the formula ∫u dv = uv - ∫v du.Choose u and dv: Choose u and dv. Let u = 5x, which implies that du = 5 dx. Let dv = e^(3x) dx, which implies that v = (1/3)e^(3x) after integrating dv with respect to x.Apply integration by parts: Apply the integration by parts formula. Using the integration by parts formula, we have: ∫5xe^(3x) dx = uv - ∫v du = (5x)(1/3)e^(3x) - ∫(1/3)e^(3x)(5 dx)Simplify and integrate: Simplify and integrate the remaining integral. = (5/3)xe^(3x) - (5/3)∫e^(3x) dx Now we integrate e^(3x) with respect to x, which gives us (1/3)e^(3x).Substitute and simplify: Substitute the integral and simplify. = (5/3)xe^(3x) - (5/3)*(1/3)e^(3x) + C = (5/3)xe^(3x) - (5/9)e^(3x) + CCombine and write final answer: Combine like terms and write the final answer. The final answer is the integral of 5xe^(3x) with respect to x: = (5/3)xe^(3x) - (5/9)e^(3x) + C

Evaluate the integral. $\newline$ $\int 5 x e^{3 x} d x$ $\newline$ Answer:

Full solution

More problems from Find indefinite integrals using the substitution and by parts

Related topics

Evaluate the integral.\newline∫5xe3xdx \int 5 x e^{3 x} d x ∫5xe3xdx\newlineAnswer:

Evaluate the integral. $\newline$ $\int 5 x e^{3 x} d x$ $\newline$ Answer: