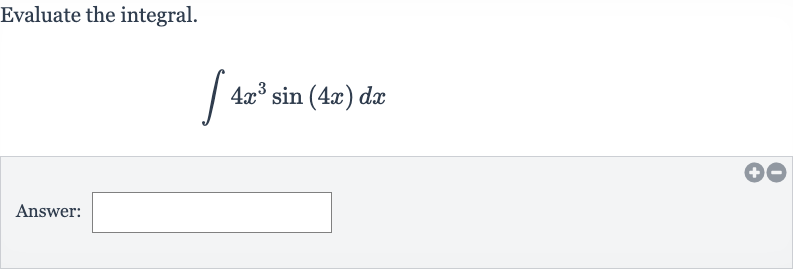
Evaluate the integral. int4x^(3)sin(4x)dx Answer:

Question

Evaluate the integral.  int4x^(3)sin(4x)dx Answer:

Bytelearn · Accepted Answer

Identify Integral: Identify the integral to be solved. We need to evaluate the integral of 4x^3sin(4x) with respect to x, which is written as ∫4x^3sin(4x)dx.Use Integration by Parts: Use integration by parts. Integration by parts is given by the formula ∫u dv = uv - ∫v du, where u and dv are parts of the integrand. We need to choose u and dv such that the resulting integral is simpler. Let's choose u = x^3 (which will simplify when differentiated) and dv = 4sin(4x)dx (which will integrate easily).Differentiate and Integrate: Differentiate u and integrate dv. Differentiating u = x^3 gives us du = 3x^2dx. Integrating dv = 4sin(4x)dx gives us v = -cos(4x).Apply Integration by Parts: Apply the integration by parts formula. Now we apply the integration by parts formula: ∫4x^3sin(4x)dx = uv - ∫v du = x^3(-cos(4x)) - ∫(-cos(4x))(3x^2)dx = -x^3cos(4x) + 3∫x^2cos(4x)dxApply Integration by Parts Again: Apply integration by parts again to the remaining integral. We need to apply integration by parts to the integral 3∫x^2cos(4x)dx. Let's choose u = x^2 and dv = 3cos(4x)dx. Differentiating u = x^2 gives us du = 2xdx. Integrating dv = 3cos(4x)dx gives us v = (3/4)sin(4x).Apply Integration by Parts Formula: Apply the integration by parts formula to the new integral. Now we apply the integration by parts formula to the new integral: 3∫x^2cos(4x)dx = uv - ∫v du = x^2(3/4)sin(4x) - ∫(3/4)sin(4x)(2x)dx = (3/4)x^2sin(4x) - (3/2)∫xsin(4x)dxApply Integration by Parts One More Time: Apply integration by parts one more time to the remaining integral. We need to apply integration by parts to the integral (3/2)∫xsin(4x)dx. Let's choose u = x and dv = (3/2)sin(4x)dx. Differentiating u = x gives us du = dx. Integrating dv = (3/2)sin(4x)dx gives us v = -(3/8)cos(4x).Apply Integration by Parts Formula: Apply the integration by parts formula to the final integral. Now we apply the integration by parts formula to the final integral: (3/2)∫xsin(4x)dx = uv - ∫v du = x(-3/8)cos(4x) - ∫(-3/8)cos(4x)dx = -(3/8)xcos(4x) + (3/8)(1/4)sin(4x) = -(3/8)xcos(4x) + (3/32)sin(4x)Combine All Parts: Combine all parts to get the final answer. Combining all parts, we get: ∫4x^3sin(4x)dx = -x^3cos(4x) + (3/4)x^2sin(4x) - (3/8)xcos(4x) + (3/32)sin(4x) + C where C is the constant of integration.

Evaluate the integral. $\newline$ $\int 4 x^{3} \sin (4 x) d x$ $\newline$ Answer:

Full solution

More problems from Find indefinite integrals using the substitution and by parts

Related topics

Evaluate the integral.\newline∫4x3sin⁡(4x)dx \int 4 x^{3} \sin (4 x) d x ∫4x3sin(4x)dx\newlineAnswer:

Evaluate the integral. $\newline$ $\int 4 x^{3} \sin (4 x) d x$ $\newline$ Answer: