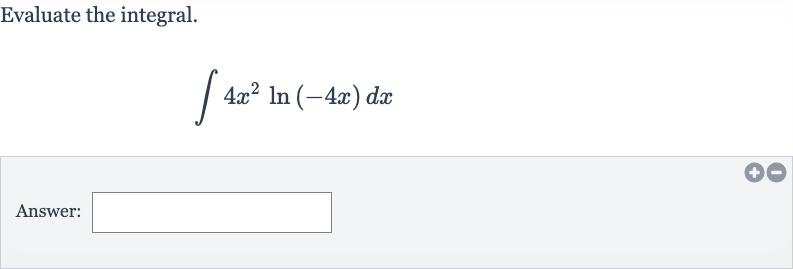
Evaluate the integral. int4x^(2)ln(-4x)dx Answer:

Question

Evaluate the integral.  int4x^(2)ln(-4x)dx Answer:

Bytelearn · Accepted Answer

Choose u and dv: Let's use integration by parts to solve the integral ∫4x^2ln(-4x)dx. Integration by parts is given by ∫udv = uv - ∫vdu, where we need to choose u and dv such that du and v are easily computable. Let's choose u = ln(-4x) and dv = 4x^2dx. Then we need to compute du and v.Compute du: First, we compute du by differentiating u with respect to x. Since u = ln(-4x), du/dx = 1/(-4x) * (-4) = -1/x. Therefore, du = -dx/x.Compute v: Next, we compute v by integrating dv. Since dv = 4x^2dx, v = ∫4x^2dx. To integrate x^2, we use the power rule ∫x^n dx = x^(n+1)/(n+1) for n ≠ -1. Thus, v = 4x^3/3.Apply integration by parts: Now we apply the integration by parts formula: ∫udv = uv - ∫vdu. Substituting u, du, v, and dv, we get ∫4x^2ln(-4x)dx = ln(-4x) * (4x^3/3) - ∫(4x^3/3) * (-dx/x).Simplify the integral: Simplify the integral on the right: ∫(4x^3/3) * (-dx/x) = -4/3 ∫x^2dx. Again, we use the power rule for integration to find ∫x^2dx = x^3/3.Perform the integration: Perform the integration: -4/3 ∫x^2dx = -4/3 * (x^3/3) = -4x^3/9.Write down full expression: Now we have all the parts to write down the full expression for the integral: ∫4x^2ln(-4x)dx = ln(-4x) * (4x^3/3) - (-4x^3/9).Simplify the expression: Simplify the expression: ln(-4x) * (4x^3/3) - (-4x^3/9) = (4x^3/3)ln(-4x) + 4x^3/9.Add constant of integration: Finally, we add the constant of integration C to our result to get the indefinite integral: ∫4x^2ln(-4x)dx = (4x^3/3)ln(-4x) + 4x^3/9 + C.

Evaluate the integral. $\newline$ $\int 4 x^{2} \ln (-4 x) d x$ $\newline$ Answer:

Full solution

More problems from Find indefinite integrals using the substitution and by parts

Related topics

Evaluate the integral.\newline∫4x2ln⁡(−4x)dx \int 4 x^{2} \ln (-4 x) d x ∫4x2ln(−4x)dx\newlineAnswer:

Evaluate the integral. $\newline$ $\int 4 x^{2} \ln (-4 x) d x$ $\newline$ Answer: