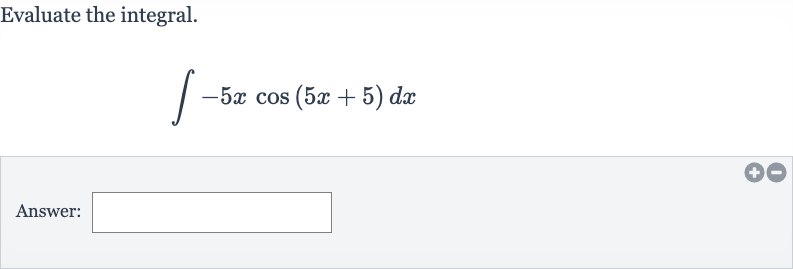
Evaluate the integral. int-5x cos(5x+5)dx Answer:

Question

Evaluate the integral.  int-5x cos(5x+5)dx Answer:

Bytelearn · Accepted Answer

Identify Integral: Let's first identify the integral we need to evaluate: I = ∫-5x cos(5x+5) dx We can use integration by parts, which states that ∫u dv = uv - ∫v du, where u is a function of x, and dv is the differential of another function of x. Let's choose u = -5x (which will be differentiated) and dv = cos(5x+5) dx (which will be integrated).Choose u and dv: Differentiate u with respect to x to find du: u = -5x du = -5 dxDifferentiate u: Integrate dv to find v: dv = cos(5x+5) dx To integrate dv, we need to use the substitution method. Let w = 5x+5, then dw = 5 dx, which means dx = dw/5. v = ∫cos(w) dw/5 v = (1/5)sin(w) + C Since w = 5x+5, we substitute back to get v in terms of x: v = (1/5)sin(5x+5)Integrate dv: Now apply the integration by parts formula: I = uv - ∫v du I = (-5x)(1/5)sin(5x+5) - ∫(1/5)sin(5x+5)(-5) dx Simplify the expression: I = -x sin(5x+5) + ∫sin(5x+5) dxApply Integration by Parts: Now we need to integrate ∫sin(5x+5) dx. We will use the substitution method again with w = 5x+5, dw = 5 dx, and dx = dw/5. I = -x sin(5x+5) + (1/5)∫sin(w) dw Integrate sin(w) with respect to w: I = -x sin(5x+5) + (1/5)(-cos(w)) + C Substitute back w = 5x+5 to get the integral in terms of x: I = -x sin(5x+5) - (1/5)cos(5x+5) + C

Evaluate the integral. $\newline$ $\int-5 x \cos (5 x+5) d x$ $\newline$ Answer:

Full solution

More problems from Find indefinite integrals using the substitution and by parts

Related topics

Evaluate the integral.\newline∫−5xcos⁡(5x+5)dx \int-5 x \cos (5 x+5) d x ∫−5xcos(5x+5)dx\newlineAnswer:

Evaluate the integral. $\newline$ $\int-5 x \cos (5 x+5) d x$ $\newline$ Answer: