AI tutor
Full solution
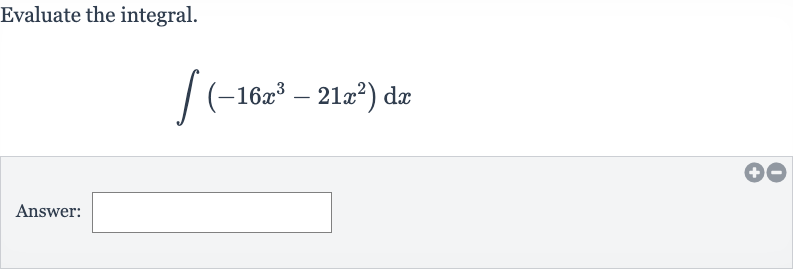
Q. Evaluate the integral.Answer:
- Apply Power Rule: Apply the power rule for integration to each term separately. The power rule states that the integral of with respect to is , where is the constant of integration.
- Integrate : Integrate the first term . Using the power rule, we add to the exponent and divide by the new exponent. Integral of
- Integrate : Integrate the second term . Using the power rule, we add to the exponent and divide by the new exponent.
- Combine Results: Combine the results of the integrals of both terms and add the constant of integration .