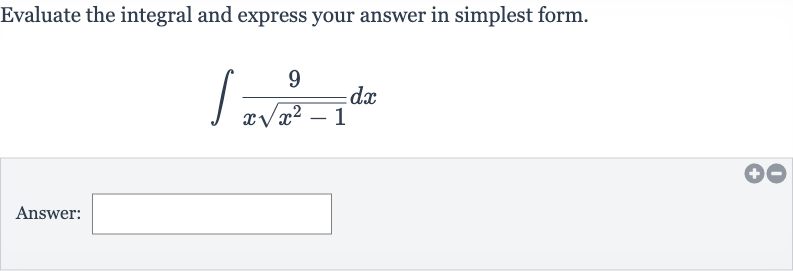
Evaluate the integral and express your answer in simplest form. int(9)/(xsqrt(x^(2)-1))dx Answer:

Question

Evaluate the integral and express your answer in simplest form.  int(9)/(xsqrt(x^(2)-1))dx Answer:

Bytelearn · Accepted Answer

Recognize standard inverse trigonometric form: Recognize the integral as a standard inverse trigonometric form. The integral resembles the form of the derivative of inverse hyperbolic functions, specifically the inverse hyperbolic secant. The standard form is int(1)/(x*sqrt(x^2-1))dx = arcsech(x) + C, where C is the constant of integration.Factor out constant: Factor out the constant from the integral. The integral can be rewritten by factoring out the constant 9: I = int(9)/(x*sqrt(x^2-1))dx = 9 * int(1)/(x*sqrt(x^2-1))dxApply standard form: Apply the standard form of the inverse hyperbolic secant. Using the standard form, we can write the integral as: I = 9 * arcsech(x) + C However, we need to express the answer in terms of the natural logarithm, as the arcsech function is not commonly used in simplest form.Express in natural logarithm: Express arcsech(x) in terms of natural logarithm. The inverse hyperbolic secant can be expressed as: arcsech(x) = ln(1/x + sqrt(1/x^2 - 1))Substitute into integral: Substitute the expression for arcsech(x) into the integral. I = 9 * ln(1/x + sqrt(1/x^2 - 1)) + CSimplify inside logarithm: Simplify the expression inside the logarithm. Since we have 1/x inside the logarithm, we can simplify the expression by multiplying the numerator and denominator by x: I = 9 * ln((1 + sqrt(x^2 - 1))/x) + CCheck for errors: Check for any possible simplifications or errors. The expression inside the logarithm is already in its simplest form, and there are no apparent math errors.

Evaluate the integral and express your answer in simplest form. $\newline$ $\int \frac{9}{x \sqrt{x^{2}-1}} d x$ $\newline$ Answer:

Full solution

More problems from Find indefinite integrals using the substitution and by parts

Related topics

Evaluate the integral and express your answer in simplest form.\newline∫9xx2−1dx \int \frac{9}{x \sqrt{x^{2}-1}} d x ∫xx2−1​9​dx\newlineAnswer:

Evaluate the integral and express your answer in simplest form. $\newline$ $\int \frac{9}{x \sqrt{x^{2}-1}} d x$ $\newline$ Answer: