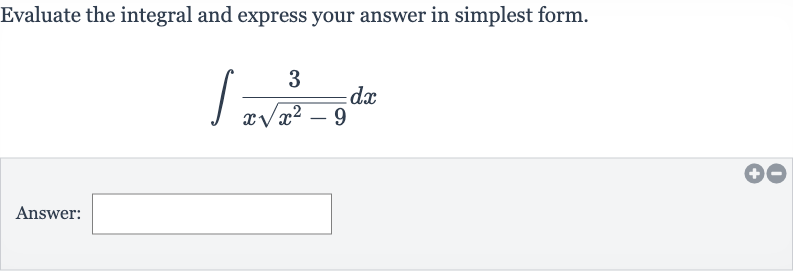
Evaluate the integral and express your answer in simplest form. int(3)/(xsqrt(x^(2)-9))dx Answer:

Question

Evaluate the integral and express your answer in simplest form.  int(3)/(xsqrt(x^(2)-9))dx Answer:

Bytelearn · Accepted Answer

Recognize standard inverse trigonometric form: Recognize the integral as a standard inverse trigonometric form. The integral resembles the form of the derivative of the inverse hyperbolic function, specifically the inverse hyperbolic secant. We can rewrite the integral in a form that matches the derivative of arcsinh(x/a) or arcsech(x/a). I = ∫(3)/(x*sqrt(x^2-9))dxUse substitution to simplify: Use substitution to simplify the integral. Let x = 3sec(θ), which implies dx = 3sec(θ)tan(θ)dθ. When x = 3sec(θ), we have sqrt(x^2 - 9) = sqrt(9sec^2(θ) - 9) = 3tan(θ). Now substitute these into the integral. I = ∫(3)/(3sec(θ)*3tan(θ)) * 3sec(θ)tan(θ)dθSimplify the expression: Simplify the expression. The 3sec(θ)tan(θ) terms cancel out, leaving us with: I = ∫(1)/tan(θ)dθRecognize natural logarithm function: Recognize the integral as the natural logarithm function. The integral of 1/tan(θ) is the natural logarithm of the absolute value of sin(θ). I = ln|sin(θ)| + C, where C is the constant of integration.Back-substitute to original variable: Back-substitute to return to the original variable x. We need to express sin(θ) in terms of x. From the substitution x = 3sec(θ), we have cos(θ) = 3/x. Since sin^2(θ) + cos^2(θ) = 1, we can solve for sin(θ) = sqrt(1 - cos^2(θ)) = sqrt(1 - (3/x)^2). I = ln|sqrt(1 - (3/x)^2)| + CSimplify expression inside logarithm: Simplify the expression inside the logarithm. We can simplify the square root and the square inside the logarithm. I = ln|sqrt(1 - 9/x^2)| + C I = ln|1/x * sqrt(x^2 - 9)| + CSimplify logarithm expression: Simplify the logarithm expression. Since ln(a*b) = ln(a) + ln(b), we can separate the terms inside the logarithm. I = ln|1/x| + ln|sqrt(x^2 - 9)| + CCombine constants into single constant: Simplify further using properties of logarithms. The natural logarithm of a square root is one-half the natural logarithm of the argument, and ln|1/x| is -ln|x|. I = -ln|x| + 1/2 * ln|x^2 - 9| + CCombine the constants into a single constant of integration. Since C is an arbitrary constant, we can combine the constants from the logarithm properties into a single constant. I = -ln|x| + 1/2 * ln|x^2 - 9| + C'

Evaluate the integral and express your answer in simplest form. $\newline$ $\int \frac{3}{x \sqrt{x^{2}-9}} d x$ $\newline$ Answer:

Full solution

More problems from Find indefinite integrals using the substitution and by parts

Related topics

Evaluate the integral and express your answer in simplest form.\newline∫3xx2−9dx \int \frac{3}{x \sqrt{x^{2}-9}} d x ∫xx2−9​3​dx\newlineAnswer:

Evaluate the integral and express your answer in simplest form. $\newline$ $\int \frac{3}{x \sqrt{x^{2}-9}} d x$ $\newline$ Answer: