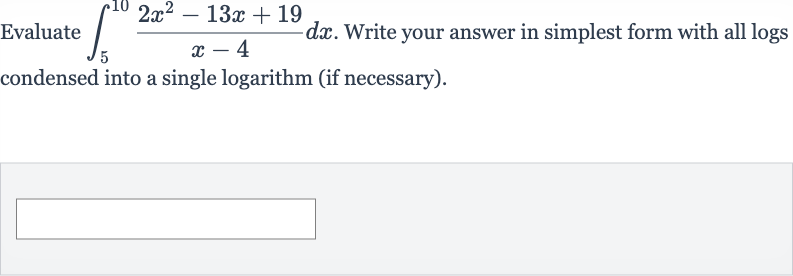
Evaluate int_(5)^(10)(2x^(2)-13 x+19)/(x-4)dx. Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).

Question

Evaluate  int_(5)^(10)(2x^(2)-13 x+19)/(x-4)dx. Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).

Bytelearn · Accepted Answer

Perform Polynomial Long Division: Perform polynomial long division to simplify the integrand. We need to divide the polynomial 2x^2 - 13x + 19 by x - 4.Polynomial Long Division Calculation: Polynomial long division calculation. 2x^2 divided by x gives 2x. Multiply (x - 4) by 2x to get 2x^2 - 8x. Subtract this from 2x^2 - 13x to get -5x. Bring down the +19 to get -5x + 19. -5x divided by x gives -5. Multiply (x - 4) by -5 to get -5x + 20. Subtract this from -5x + 19 to get -1. The result of the division is 2x - 5 with a remainder of -1.Rewrite Integral with Result: Rewrite the integral with the result of the division. The integral becomes: ∫(2x - 5)dx - ∫(1/(x - 4))dx, evaluated from 5 to 10.Integrate Polynomial Part: Integrate the polynomial part. The integral of 2x - 5 with respect to x is x^2 - 5x.Integrate Rational Part: Integrate the rational part. The integral of 1/(x - 4) with respect to x is ln|x - 4|.Combine and Evaluate Results: Combine the results and evaluate from 5 to 10. The integral becomes: (x^2 - 5x) - ln|x - 4|, evaluated from 5 to 10.Evaluate at Upper Limit: Evaluate the antiderivative at the upper limit x = 10. Substitute x = 10 into the antiderivative to get: (10^2 - 5*10) - ln|10 - 4| = (100 - 50) - ln|6| = 50 - ln(6).Evaluate at Lower Limit: Evaluate the antiderivative at the lower limit x = 5. Substitute x = 5 into the antiderivative to get: (5^2 - 5*5) - ln|5 - 4| = (25 - 25) - ln|1| = 0 - ln(1) = 0.Subtract Lower from Upper: Subtract the value at the lower limit from the value at the upper limit. (50 - ln(6)) - (0) = 50 - ln(6).Write Final Answer: Write the final answer in simplest form. The final answer is 50 - ln(6).

Evaluate $\int_{5}^{10} \frac{2 x^{2}-13 x+19}{x-4} d x$ . Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).

Full solution

More problems from Find indefinite integrals using the substitution

Related topics

Evaluate ∫5102x2−13x+19x−4dx \int_{5}^{10} \frac{2 x^{2}-13 x+19}{x-4} d x ∫510​x−42x2−13x+19​dx. Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).

Evaluate $\int_{5}^{10} \frac{2 x^{2}-13 x+19}{x-4} d x$ . Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).