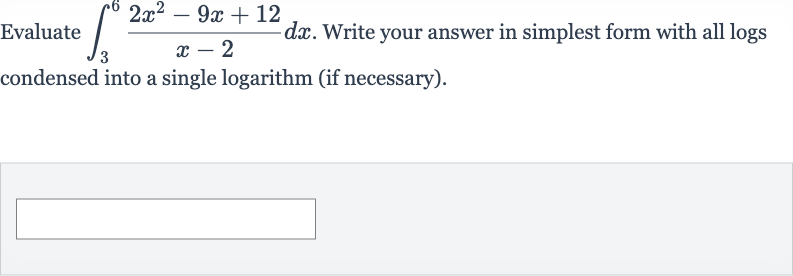
Evaluate int_(3)^(6)(2x^(2)-9x+12)/(x-2)dx. Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).

Question

Evaluate  int_(3)^(6)(2x^(2)-9x+12)/(x-2)dx. Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).

Bytelearn · Accepted Answer

Perform Polynomial Division: First, we need to perform polynomial long division or synthetic division to simplify the integrand (2x^2 - 9x + 12) / (x - 2) since the numerator is a polynomial of higher degree than the denominator.Integrate Simplified Function: Performing the polynomial long division, we divide 2x^2 - 9x + 12 by x - 2:              2x - 5           ___________ x - 2 | 2x^2 - 9x + 12          - (2x^2 - 4x)           ___________                -5x + 12                - (-5x + 10)           ___________                      2  The result of the division is 2x - 5 with a remainder of 2. So, the integrand can be rewritten as: (2x^2 - 9x + 12) / (x - 2) = 2x - 5 + 2/(x - 2)Evaluate Definite Integral: Now we can integrate the function term by term: ∫(2x - 5 + 2/(x - 2))dx = ∫2xdx - ∫5dx + ∫2/(x - 2)dxSubstitute Values: Integrating each term gives us: ∫2xdx = x^2 ∫5dx = 5x ∫2/(x - 2)dx = 2ln|x - 2|  So the indefinite integral is: x^2 - 5x + 2ln|x - 2| + CSimplify Final Answer: Now we need to evaluate the definite integral from x = 3 to x = 6. We do this by substituting the upper and lower limits into the indefinite integral and finding the difference: F(6) - F(3) = [6^2 - 5(6) + 2ln|6 - 2|] - [3^2 - 5(3) + 2ln|3 - 2|]Substitute the values and simplify: F(6) - F(3) = [36 - 30 + 2ln4] - [9 - 15 + 2ln1] = [6 + 2ln4] - [-6 + 0] = 6 + 2ln4 + 6 = 12 + 2ln4Since ln1 is 0, we can simplify further: 12 + 2ln4 = 12 + 2ln(2^2) = 12 + 4ln2The final answer in simplest form with all logs condensed into a single logarithm is: 12 + 4ln2

Evaluate $\int_{3}^{6} \frac{2 x^{2}-9 x+12}{x-2} d x$ . Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).

Full solution

More problems from Find indefinite integrals using the substitution

Related topics

Evaluate ∫362x2−9x+12x−2dx \int_{3}^{6} \frac{2 x^{2}-9 x+12}{x-2} d x ∫36​x−22x2−9x+12​dx. Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).

Evaluate $\int_{3}^{6} \frac{2 x^{2}-9 x+12}{x-2} d x$ . Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).