Full solution
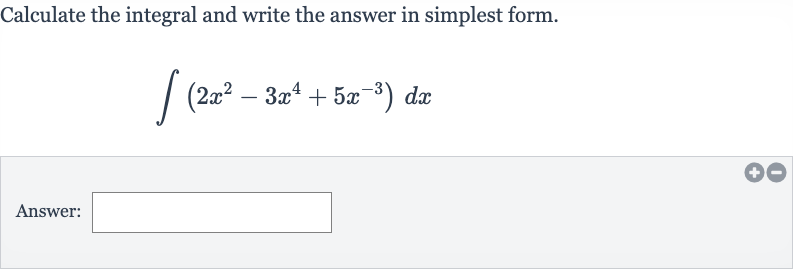
Q. Calculate the integral and write the answer in simplest form.Answer:
- Given Integral: We are given the integral of a polynomial function: To solve this, we will integrate each term separately using the power rule for integration, which states that the integral of with respect to is for .
- Integrating : First, we integrate the term :
- Integrating : Next, we integrate the term :
- Integrating : Finally, we integrate the term :
- Combining Integrated Terms: Now, we combine all the integrated terms and add the constant of integration :
Integral =