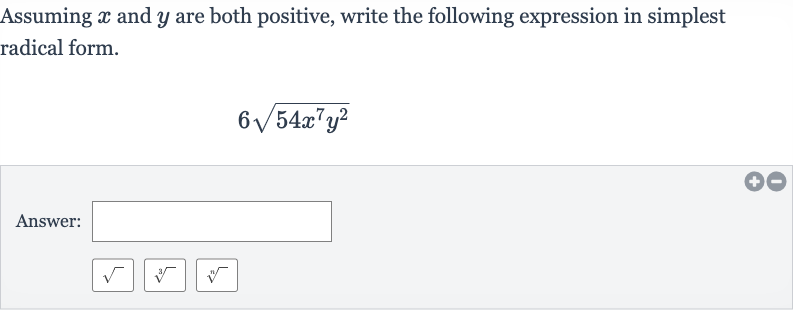
Assuming x and y are both positive, write the following expression in simplest radical form. 6sqrt(54x^(7)y^(2)) Answer:

Question

Assuming  x and  y are both positive, write the following expression in simplest radical form.  6sqrt(54x^(7)y^(2)) Answer:

Bytelearn · Accepted Answer

Factorize 54: We need to simplify the expression 6sqrt(54x^(7)y^(2)). Let's start by factoring the number 54 into its prime factors and simplifying the expression inside the square root. 54 can be factored into 2 * 27, and 27 is 3^3. So we can rewrite 54 as 2 * 3^3.Express factors separately: Now we can express the square root of each factor separately: 6sqrt(54x^(7)y^(2)) = 6sqrt(2 * 3^3 * x^(7) * y^(2)).Take out perfect squares: We can take out the square root of any perfect squares from under the radical. Since 3^3 contains a perfect square, which is 3^2, and x^7 contains a perfect square, which is x^6, and y^2 is already a perfect square, we can simplify further: 6sqrt(2 * 3^3 * x^(7) * y^(2)) = 6 * 3 * x^3 * y * sqrt(2 * 3 * x).Multiply constants and variables: Now we multiply the constants and the variables that are outside the square root: 6 * 3 * x^3 * y = 18x^3y. So the expression becomes: 18x^3y * sqrt(2 * 3 * x).Simplify expression inside square root: Finally, we can simplify the expression inside the square root by combining the constants: sqrt(2 * 3 * x) = sqrt(6x). So the entire expression simplifies to: 18x^3y * sqrt(6x).

Assuming $x$ and $y$ are both positive, write the following expression in simplest radical form. $\newline$ $6 \sqrt{54 x^{7} y^{2}}$ $\newline$ Answer:

Full solution

More problems from Find indefinite integrals using the substitution

Related topics

Assuming x x x and y y y are both positive, write the following expression in simplest radical form.\newline654x7y2 6 \sqrt{54 x^{7} y^{2}} 654x7y2​\newlineAnswer:

Assuming $x$ and $y$ are both positive, write the following expression in simplest radical form. $\newline$ $6 \sqrt{54 x^{7} y^{2}}$ $\newline$ Answer: