Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Assuming
x and
y are both positive, write the following expression in simplest radical form.

2sqrt(25x^(6)y^(5))
Answer:

Assuming $x$ and $y$ are both positive, write the following expression in simplest radical form. $\newline$ $2 \sqrt{25 x^{6} y^{5}}$ $\newline$ Answer:

View step-by-step help

View step-by-step help

Find indefinite integrals using the substitution

Full solution

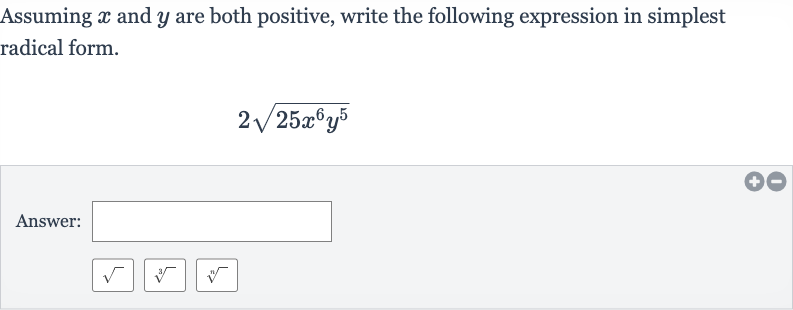
Q. Assuming

x

and

y

are both positive, write the following expression in simplest radical form.

\newline

2 \sqrt{25 x^{6} y^{5}}

\newline

Answer:

Simplify Product Inside Radical: We start by simplifying the square root of the product inside the radical. We know that the square root of a product is the product of the square roots, and we can also simplify the square root of a perfect square. $\newline$ $2\sqrt{25x^{6}y^{5}} = 2\sqrt{25} \times \sqrt{x^{6}} \times \sqrt{y^{5}}$
Simplify Square Roots: Next, we simplify each square root separately. The square root of $25$ is $5$ , the square root of $x^6$ is $x^3$ because $(x^3)^2 = x^6$ , and the square root of $y^5$ is $y^2 \sqrt{y}$ because $(y^2)^2 \cdot y = y^5$ . $\newline$ $2 \cdot 5 \cdot x^3 \cdot y^2 \cdot \sqrt{y}$
Multiply Constants and Like Terms: Now we multiply the constants and the like terms together. $2 \times 5 = 10$ $10 \times x^3 \times y^2 \times \sqrt{y}$
Write in Simplest Form: Finally, we write the expression in its simplest radical form. $10x^3y^2\sqrt{y}$

More problems from Find indefinite integrals using the substitution

Question

Evaluate the integral.

\newline

\int-x 4^{-3 x} d x

\newline

Posted 9 months ago

Question

Evaluate the integral.

\newline

\int 6 x^{3} e^{-2 x} d x

\newline

Posted 9 months ago

Question

Evaluate the integral.

\newline

\int x 5^{3 x} d x

\newline

Posted 9 months ago

Question

Evaluate the integral.

\newline

\int-2 x e^{2 x} d x

\newline

Posted 9 months ago

Question

Evaluate the integral.

\newline

\int-2 x \cos (-2 x) d x

\newline

Posted 9 months ago

Question

Evaluate the integral.

\newline

\int-x \cos (-3 x) d x

\newline

Posted 9 months ago

Question

Evaluate the integral.

\newline

\int 6 x^{2} 5^{3 x} d x

\newline

Posted 9 months ago

Question

Evaluate the integral.

\newline

\int-6 x 4^{-4 x} d x

\newline

Posted 9 months ago

Question

Evaluate the integral.

\newline

\int 6 x^{2} 2^{3 x} d x

\newline

Posted 9 months ago

Question

Evaluate the integral.

\newline

\int-3 x \sin (-2 x) d x

\newline

Posted 9 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant