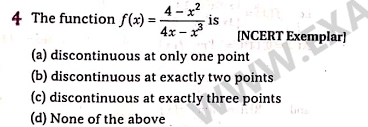4 The function f(x)=(4-x^(2))/(4x-x^(3)) is (a) discontinuous at only one point (b) discontinuous at exactly two points (c) discontinuous at exactly three points (d) None of the above

Question

4 The function  f(x)=(4-x^(2))/(4x-x^(3)) is  (a) discontinuous at only one point (b) discontinuous at exactly two points (c) discontinuous at exactly three points (d) None of the above

Bytelearn · Accepted Answer

Factorize Denominator: To find the points of discontinuity, we need to determine the values of x for which the denominator of the function becomes zero, as these will be the points where the function is undefined. The denominator is 4x - x^3. We can factor out an x to simplify it: 4x - x^3 = x(4 - x^2)Set Denominator Equal to Zero: Now we set the factored denominator equal to zero to find the values of x that cause the function to be undefined: x(4 - x^2) = 0 This gives us two equations to solve: 1) x = 0 2) 4 - x^2 = 0Solve for x=0: Solving the first equation is straightforward: x = 0 This is our first point of discontinuity.Solve for x=2 and x=-2: Solving the second equation: 4 - x^2 = 0 x^2 = 4 Taking the square root of both sides gives us two solutions: x = 2 and x = -2 These are our second and third points of discontinuity.Final Discontinuity Points: We have found three points of discontinuity for the function f(x)=(4-x^2)/(4x-x^3), which are x = 0, x = 2, and x = -2. Therefore, the function is discontinuous at exactly three points.

The function $\newline$ $f(x)=\frac{4-x^{2}}{4x-x^{3}}$ is $\newline$ (a) discontinuous at only one point $\newline$ (b) discontinuous at exactly two points $\newline$ (c) discontinuous at exactly three points $\newline$ (d) None of the above

Full solution

More problems from Find indefinite integrals using the substitution

Related topics

The function \newlinef(x)=4−x24x−x3f(x)=\frac{4-x^{2}}{4x-x^{3}}f(x)=4x−x34−x2​ is\newline(a) discontinuous at only one point\newline(b) discontinuous at exactly two points\newline(c) discontinuous at exactly three points\newline(d) None of the above

The function $\newline$ $f(x)=\frac{4-x^{2}}{4x-x^{3}}$ is $\newline$ (a) discontinuous at only one point $\newline$ (b) discontinuous at exactly two points $\newline$ (c) discontinuous at exactly three points $\newline$ (d) None of the above