Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

(dy)/(dt)=2t+3 and y(1)=6.
What is t when y=0 ?
Choose all answers that apply:
(A) t=-3
(B) t=-1
(C) t=1
(D) t=0
(E) t=-2
(F) t=-4

$\frac{d y}{d t}=2 t+3 \text { and } y(1)=6 .$ $\newline$ What is $t$ when $y=0$ ? $\newline$ Choose all answers that apply: $\newline$ (A) $t=-3$ $\newline$ (B) $t=-1$ $\newline$ (C) $t=1$ $\newline$ (D) $t=0$ $\newline$ (E) $t=-2$ $\newline$ (F) $t=-4$

View step-by-step help

View step-by-step help

Full solution

Q.

\frac{d y}{d t}=2 t+3 \text { and } y(1)=6 .

\newline

What is

t

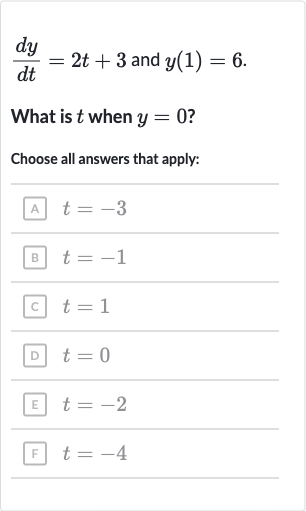
when

y=0

?

\newline

Choose all answers that apply:

\newline

(A)

t=-3

\newline

(B)

t=-1

\newline

(C)

t=1

\newline

(D)

t=0

\newline

(E)

t=-2

\newline

(F)

t=-4

Given Differential Equation: Given the differential equation $\frac{dy}{dt} = 2t + 3$ and the initial condition $y(1) = 6$ , we want to find the value of $t$ when $y = 0$ . To do this, we will separate variables and integrate both sides of the differential equation.
Separate Variables and Integrate: Separate the variables by moving all terms involving $y$ to one side and all terms involving $t$ to the other side. Since there are no $y$ terms on the right side, we can directly integrate with respect to $t$ .
Use Initial Condition: Integrate both sides of the equation with respect to $t$ . The integral of $\frac{dy}{dt}$ with respect to $t$ is $y$ , and the integral of $2t + 3$ with respect to $t$ is $t^2 + 3t + C$ , where $C$ is the constant of integration. $\newline$ $\int(dy) = \int(2t + 3)dt$ $\newline$ $y = t^2 + 3t + C$
Find Constant C: Use the initial condition $y(1) = 6$ to find the value of the constant $C$ . $6 = (1)^2 + 3(1) + C$ $6 = 1 + 3 + C$ $C = 6 - 4$ $C = 2$
Write General Solution: Now that we have the constant $C$ , we can write the general solution to the differential equation as: $\newline$ $y = t^2 + 3t + 2$
Find Value of t: To find the value of $t$ when $y = 0$ , we set the equation $y = t^2 + 3t + 2$ equal to $0$ and solve for $t$ . $0 = t^2 + 3t + 2$
Factor Quadratic Equation: Factor the quadratic equation to find the values of $t$ . $0 = (t + 1)(t + 2)$ This gives us two possible solutions for $t$ : $t = -1$ and $t = -2$ .
Check Answer Choices: Check the answer choices to see which ones match our solutions. The correct answers are: $\newline$ B $t = -1$ $\newline$ E $t = -2$

More problems from Euler's method

Question

f(x)=x^{3}-6 x^{2}+12 x

c

be the number that satisfies the Mean Value Theorem for

f

on the interval

[0,3]

\newline

c

\newline

1

\newline

0

\newline

1

\newline

2

\newline

3

Posted 2 months ago

Question

g(x)=x^{3}+12 x^{2}+36 x

c

be the number that satisfies the Mean Value Theorem for

g

on the interval

-8 \leq x \leq-2

\newline

c

\newline

1

\newline

-7

\newline

-6

\newline

-3

\newline

-1

Posted 2 months ago

Question

h(x)=x^{3}-6 x^{2}-10 x

c

be the number that satisfies the Mean Value Theorem for

h

on the interval

[-4,5]

\newline

c

\newline

1

\newline

-2

\newline

-1

\newline

1

\newline

3

Posted 1 month ago

Question

g(x)=2 x^{3}-21 x^{2}+60 x

\newline

What is the absolute maximum value of

g

over the closed interval

[0,6]

\newline

1

\newline

25

\newline

42

\newline

36

\newline

52

Posted 1 month ago

Question

f(x)=2 x^{3}+21 x^{2}+36 x

\newline

What is the absolute maximum value of

f

over the closed interval

[-8,0]

\newline

1

\newline

32

\newline

\mathbf{1 8 0}

\newline

\mathbf{1 0 8}

\newline

0

Posted 2 months ago

Question

h(x)=x^{3}+6 x^{2}+2

\newline

What is the absolute minimum value of

h

over the closed interval

-6 \leq x \leq 2

\newline

1

\newline

34

\newline

-34

\newline

2

\newline

-2

Posted 2 months ago

Question

1,000=20z^{2}

\newline

How many distinct real solutions does the given equation have?

\newline

1

\newline

0

\newline

1

\newline

2

\newline

4

Posted 2 months ago

Question

Dante commutes to work

4

mornings a week. For his commute each morning, he walks for

10

minutes, waits and rides the bus for

x

minutes, and waits and rides the train for

y

minutes. If Dante spends at least

3.5

hours on his morning commute each week, which of the following inequalities best describes Dante's weekly morning commute?

\newline

1

\newline

x+y+10 \geq 3.5(60)

\newline

x+y+10 \geq 3.5(60)(4)

\newline

4(x+y)+10 \geq 3.5(60)

\newline

4(x+y+10) \geq 3.5(60)

Posted 2 months ago

Question

\frac{1}{3}x+3y=4

\newline

2x-4=2y

\newline

Consider the given system of equations. If

(x,y)

is the solution to the system, then what is the value of

x-y

\newline

\square

Posted 1 month ago

Question

2

\frac{x}{2}-4=\frac{x}{3}+2

\newline

a) List the title for this type of problem(

10

\newline

b) List the section where you would find nc

\newline

c) List the procedure or rules written in yo

Posted 2 months ago