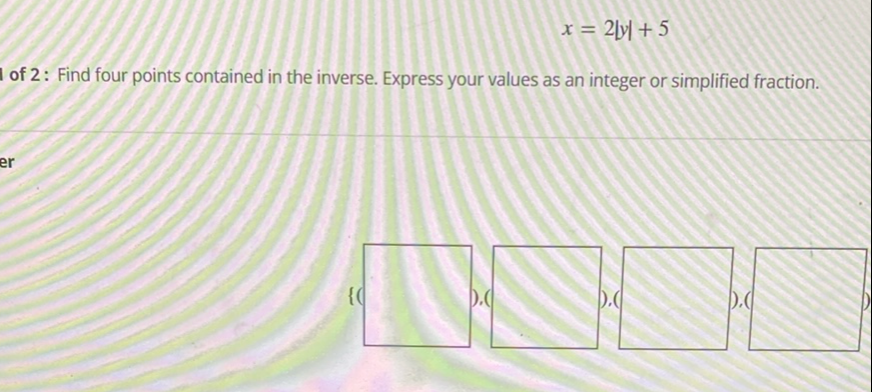
x=2|y|+5 Find four points contained in the inverse. Express your values as an integer or simplified fraction.

Question

Bytelearn · Accepted Answer

Express y in terms of x: To find the inverse of the function, we first need to express y in terms of x. This means we need to solve the equation for y.Isolate y term: Subtract 5 from both sides of the equation to isolate the term with y on one side. x - 5 = 2|y|Solve for |y|: Now, divide both sides by 2 to solve for |y|. (x - 5) / 2 = |y|Consider two cases: Since |y| represents the absolute value of y, it means that y can be either positive or negative, but not both at the same time. Therefore, we have two cases to consider: Case 1: y = (x - 5) / 2 Case 2: y = -(x - 5) / 2Find points for inverse: Now we need to find four points that are contained in the inverse. We can choose arbitrary values for x and solve for y in both cases. Let's choose x = 7, x = 9, x = 11, and x = 13 as our x-values.Point 1: x=7: For x = 7: Case 1: y = (7 - 5) / 2 = 2 / 2 = 1 Case 2: y = -(7 - 5) / 2 = -2 / 2 = -1 So, the points are (7, 1) and (7, -1).Point 2: x=9: For x = 9: Case 1: y = (9 - 5) / 2 = 4 / 2 = 2 Case 2: y = -(9 - 5) / 2 = -4 / 2 = -2 So, the points are (9, 2) and (9, -2).Point 3: x=11: For x = 11: Case 1: y = (11 - 5) / 2 = 6 / 2 = 3 Case 2: y = -(11 - 5) / 2 = -6 / 2 = -3 So, the points are (11, 3) and (11, -3).Point 4: x=13: For x = 13: Case 1: y = (13 - 5) / 2 = 8 / 2 = 4 Case 2: y = -(13 - 5) / 2 = -8 / 2 = -4 So, the points are (13, 4) and (13, -4).

$x=2|y|+5$ $\newline$ Find four points contained in the inverse. Express your values as an integer or simplified fraction.

Full solution

More problems from Solve absolute value equations

Related topics

x=2∣y∣+5x=2|y|+5x=2∣y∣+5\newlineFind four points contained in the inverse. Express your values as an integer or simplified fraction.

$x=2|y|+5$ $\newline$ Find four points contained in the inverse. Express your values as an integer or simplified fraction.