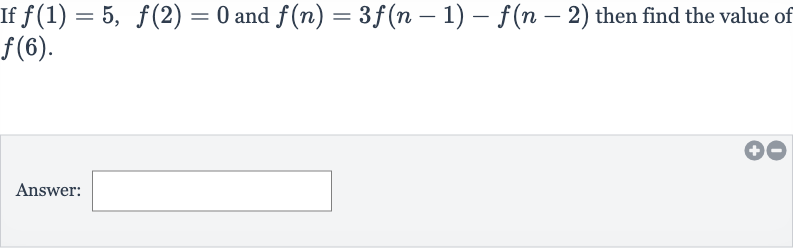AI tutor
Full solution
Q. If and then find the value of .Answer:
- Find : Use the given recursive formula to find . The recursive formula is . We know and . Calculate using the formula. .
- Find : Use the recursive formula to find . We now know and . Calculate using the formula. .
- Find : Use the recursive formula to find . We now know and . Calculate using the formula. .
- Find : Use the recursive formula to find . We now know and . Calculate using the formula. .
More problems from Write and solve direct variation equations
Question
In the given equation, is a constant. If the equation has the solutions and , what is the value of ?
Get tutor helpQuestion
In the given equation, is a constant. If the equation has the solutions and , what is the value of ?
Get tutor helpQuestion
In the given equation, is a constant. If the equation has the solutions and , what is the value of ?
Get tutor helpQuestionGet tutor help
QuestionGet tutor help