Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Given that f(x)=4x,g(x)=x-1 and h(x)=2f(x+3)-2g(x), then what is the value of h(6) ? Answer:

Given that f(x)=4x,g(x)=x−1 f(x)=4 x, g(x)=x-1 and h(x)=2f(x+3)−2g(x) h(x)=2 f(x+3)-2 g(x) , then what is the value of h(6) h(6) ?\newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Grade 8right chevron icon
Write and solve direct variation equationsright chevron icon

Full solution

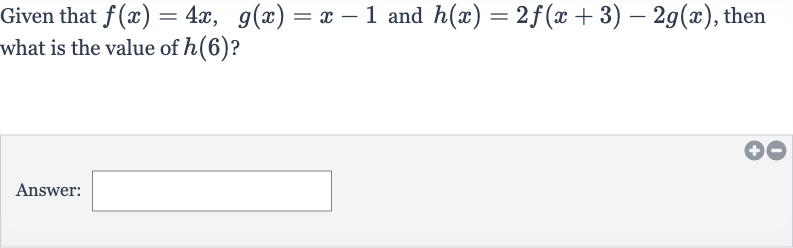
Q. Given that f(x)=4x,g(x)=x−1 f(x)=4 x, g(x)=x-1 and h(x)=2f(x+3)−2g(x) h(x)=2 f(x+3)-2 g(x) , then what is the value of h(6) h(6) ?\newlineAnswer:
  1. Define h(x)h(x): Define the function h(x)h(x) in terms of f(x)f(x) and g(x)g(x).\newlineh(x)=2f(x+3)−2g(x)h(x) = 2f(x+3) - 2g(x)\newlineWe need to find the value of h(6)h(6), so we will substitute xx with 66 in the functions f(x)f(x) and g(x)g(x) later on.
  2. Calculate f(x+3)f(x+3): Calculate f(x+3)f(x+3) when x=6x = 6.\newlinef(x)=4xf(x) = 4x, so f(x+3)=4(x+3)f(x+3) = 4(x+3).\newlineNow substitute xx with 66.\newlinef(6+3)=4(6+3)=4(9)=36f(6+3) = 4(6+3) = 4(9) = 36.
  3. Calculate g(x)g(x): Calculate g(x)g(x) when x=6x = 6.\newlineg(x)=x−1g(x) = x - 1, so g(6)=6−1=5g(6) = 6 - 1 = 5.
  4. Substitute values into h(x)h(x): Substitute the values of f(x+3)f(x+3) and g(x)g(x) into h(x)h(x).
    h(x)=2f(x+3)−2g(x)h(x) = 2f(x+3) - 2g(x).
    Now substitute f(6+3)f(6+3) and g(6)g(6) into h(6)h(6).
    h(6)=2f(6+3)−2g(6)=2(36)−2(5)=72−10=62h(6) = 2f(6+3) - 2g(6) = 2(36) - 2(5) = 72 - 10 = 62.

More problems from Write and solve direct variation equations

Question
(x−3)(ax+4)=0 (x-3)(a x+4)=0 \newlineIn the given equation, a a is a constant. If the equation has the solutions x=3 x=3 and x=−2 x=-2 , what is the value of a a ?
Get tutor helpright-arrow

Posted 8 months ago

Question
3x2+ax−5=0 3 x^{2}+a x-5=0 \newlineIn the given equation, a a is a constant. If the equation has the solutions x=−5 x=-5 and x=13 x=\frac{1}{3} , what is the value of a a ?
Get tutor helpright-arrow

Posted 7 months ago

Question
ax2+5x+2=0 a x^{2}+5 x+2=0 \newlineIn the given equation, a a is a constant. If the equation has the solutions x=−2 x=-2 and x=−12 x=-\frac{1}{2} , what is the value of a a ?
Get tutor helpright-arrow

Posted 8 months ago

Question
Find the average value of the function f(x)=2x−11 f(x)=\frac{2}{x-11} from x=2 x=2 to x=8 x=8 . Express your answer as a constant times ln⁡3 \ln 3 .\newlineAnswer: □ln⁡3\square \ln 3
Get tutor helpright-arrow

Posted 9 months ago

Question
evaluate 6+4a+b36+\frac{4}{a}+\frac{b}{3} when a=4a=4 and b=3b=3
Get tutor helpright-arrow

Posted 3 months ago

Question
4x−32kx=53 4 x-32 k x=53 \newlineIn the given equation, k k is a constant. The equation has no solution. What is the value of k k ?
Get tutor helpright-arrow

Posted 8 months ago

Question
If a1=9 a_{1}=9 and an+1=2an+2 a_{n+1}=2 a_{n}+2 then find the value of a5 a_{5} .\newlineAnswer:
Get tutor helpright-arrow

Posted 9 months ago

Question
If a1=4 a_{1}=4 and an=5an−1−n a_{n}=5 a_{n-1}-n then find the value of a5 a_{5} .\newlineAnswer:
Get tutor helpright-arrow

Posted 9 months ago

Question
If a1=1 a_{1}=1 and an=nan−1+4 a_{n}=n a_{n-1}+4 then find the value of a5 a_{5} .\newlineAnswer:
Get tutor helpright-arrow

Posted 9 months ago

Question
If a1=9 a_{1}=9 and an=nan−1−2 a_{n}=n a_{n-1}-2 then find the value of a5 a_{5} .\newlineAnswer:
Get tutor helpright-arrow

Posted 9 months ago

View all (71)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant