AI tutor
Full solution
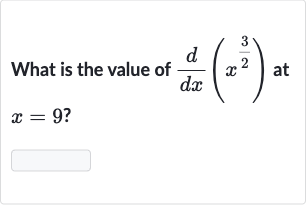
Q. What is the value of at ?
- Apply Power Rule: We need to find the derivative of the function with respect to . To do this, we will use the power rule for differentiation, which states that if , then .
- Calculate Derivative: Applying the power rule to our function, we get . Simplifying the exponent, we have .
- Substitute : Now we need to evaluate the derivative at . We substitute with in the derivative to get .
- Simplify Exponent: To simplify , we recognize that it is the square root of , which is . So, .
- Final Derivative Value: Multiplying by , we get or .
More problems from Evaluate rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help