Full solution
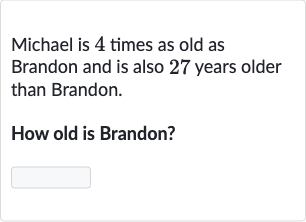
Q. Michael is \(4\) times as old as Brandon and is also \(27\) years older than Brandon.How old is Brandon?
- Equation : Michael's age in terms of Brandon's age: Let's denote Brandon's age as and Michael's age as . According to the problem, Michael is times as old as Brandon, which gives us the first equation:
- Equation : Michael's age in terms of Brandon's age and the age difference: The problem also states that Michael is years older than Brandon, which gives us the second equation:
- System of Equations: Now we have a system of two equations:) ) We can set these two equations equal to each other since they both equal .
- Setting the Equations Equal: Next, we solve for by subtracting from both sides of the equation:
- Solving for Brandon's Age: Now, we divide both sides by to find the value of B:
More problems from Solve a system of equations using any method: word problems
QuestionGet tutor help
QuestionGet tutor help