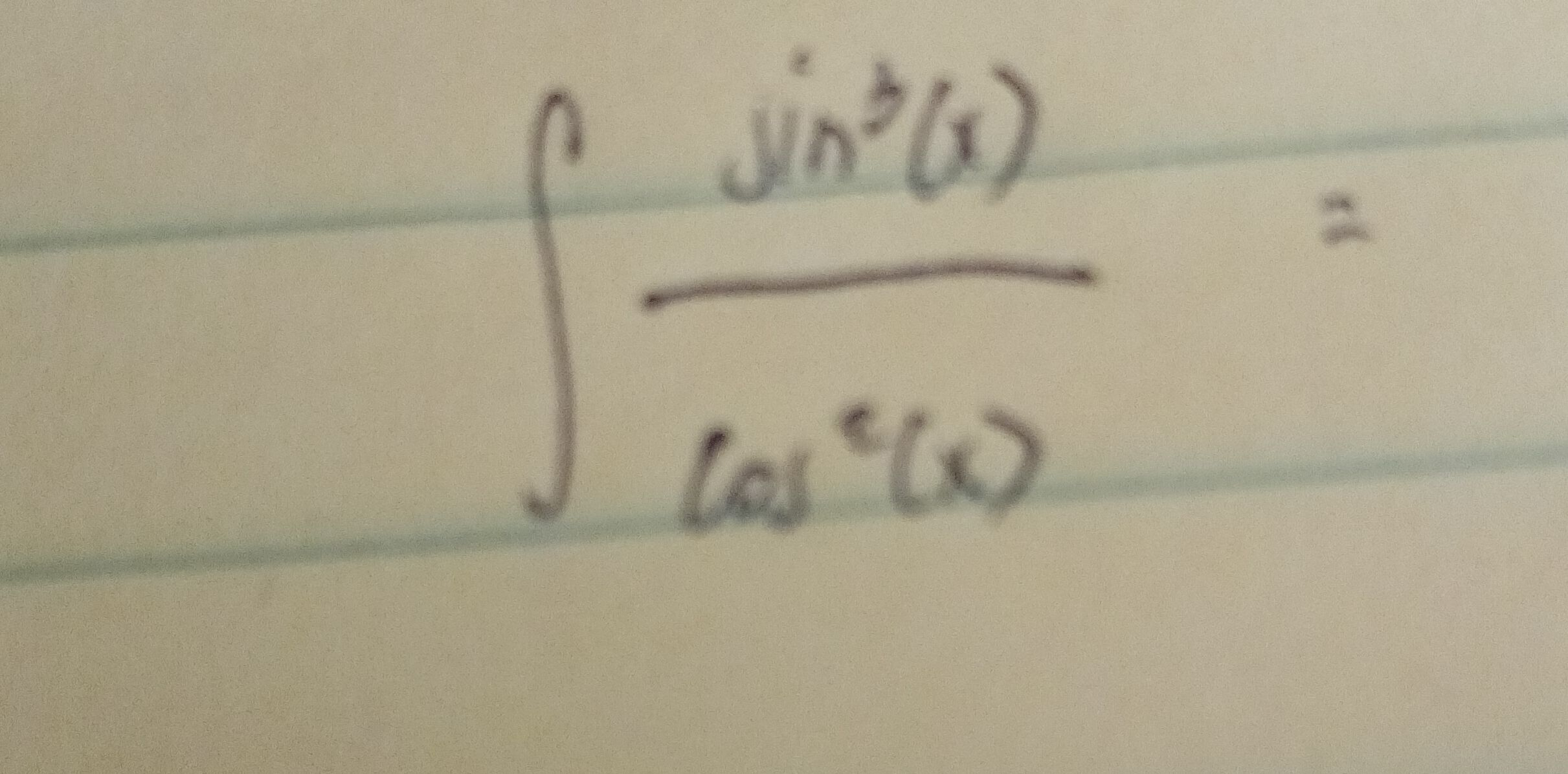AI tutor
Full solution
Q.
- Rewrite Trig Functions: Rewrite the integral in terms of sine and cosine to make it easier to integrate.The integral is already in terms of sine and cosine, so no rewriting is necessary.
- Use Trig Identity: Recognize that and rewrite the integral using this identity.
- Expand Integrand: Expand the integrand to separate the terms. -
- Simplify Integral: Simplify the integral.
- Recognize Integrals: Recognize that the first integral is the integral of and the second integral is the integral of .
- Integrate Separately: Integrate and separately.The integral of is , and the integral of is .
- Combine Results: Combine the results to get the final answer.