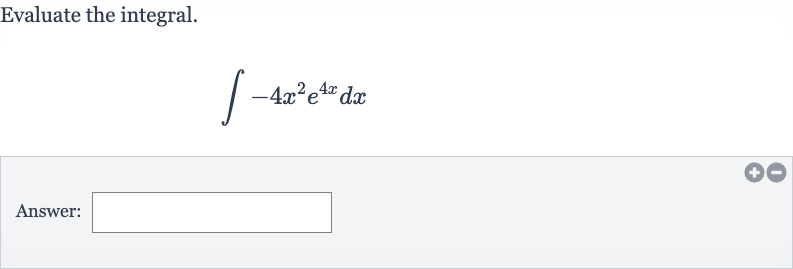AI tutor
Full solution
Q. Evaluate the integral.Answer:
- Identify integral: Identify the integral that needs to be solved.We need to find the indefinite integral of the function with respect to .
- Use integration by parts: Use integration by parts.Integration by parts formula is .Let (which implies ) and (which implies ).
- Differentiate and integrate: Differentiate and integrate . Differentiating gives us . Integrating gives us
- Apply integration by parts: Apply the integration by parts formula.= =
- Apply integration by parts again: Apply integration by parts again to the remaining integral.For the integral , let (which implies ) and (which implies ).
- Differentiate and integrate: Differentiate and integrate for the second application of integration by parts.Differentiating gives us .Integrating gives us .
- Apply integration by parts: Apply the integration by parts formula to the remaining integral.= =
- Substitute and simplify: Substitute the result from Step back into the equation from Step .
- Substitute and simplify: Substitute the result from Step back into the equation from Step .= Combine the constants and simplify the expression.The final answer is the indefinite integral of the given function: