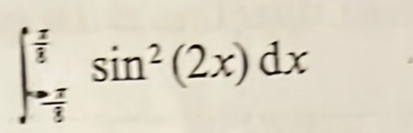AI tutor
Full solution
Q.
Evaluate the integral:
- Apply power-reduction formula: Use the power-reduction formula for sine, which states that . This will simplify the integral.The integral becomes:
- Split into two integrals: Split the integral into two separate integrals. \int_{(-\pi)/(\(8\))}^{(\pi)/(\(8\))}(\frac{\(1\)}{\(2\)} - \frac{\(1\)}{\(2\)} \cos(\(4x))dx = \frac{}{} \int_{(-\pi)/()}^{(\pi)/()}dx - \frac{}{} \int_{(-\pi)/()}^{(\pi)/()}\cos(x)dx
- Evaluate constant integral: Evaluate the first integral, which is a constant with respect to .
- Integrate cosine function: Evaluate the second integral, which involves the cosine function. To integrate , we use the substitution , which gives us or .
- Change limits with substitution: Change the limits of integration according to the substitution .When , .When , .The integral becomes:
- Evaluate integral of cosine: Evaluate the integral of from to .
- Combine results for final answer: Combine the results from Step and Step to get the final answer.The integral of from to is:
- Simplify final answer: Simplify the final answer.