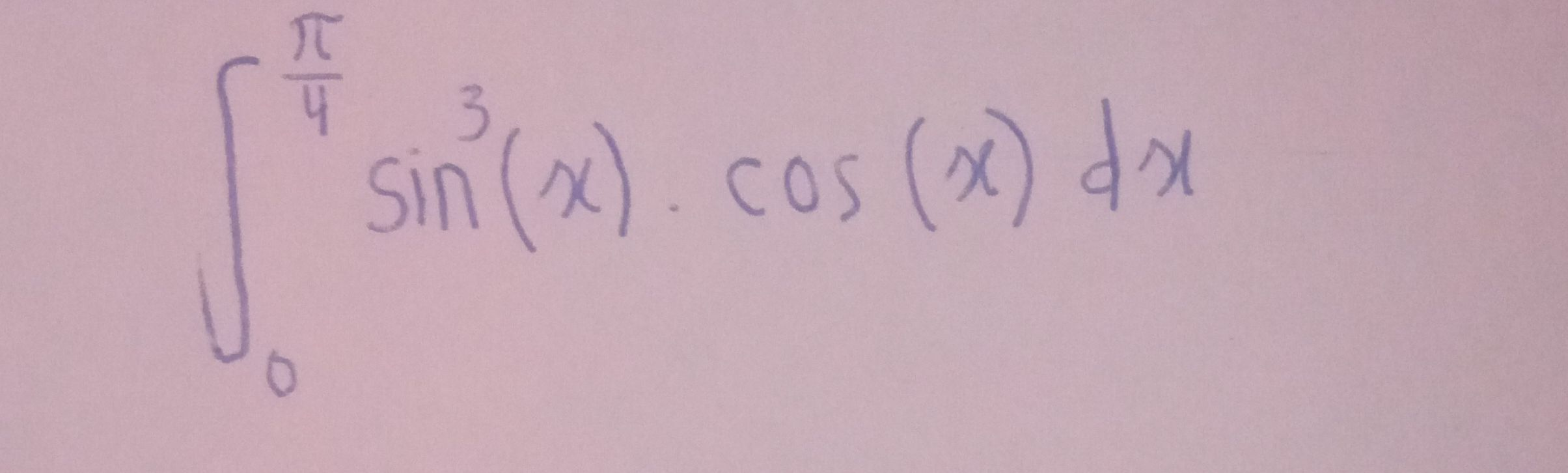AI tutor
Full solution
Q.
- Given Integral: We are given the integral to evaluate: To solve this integral, we can use the substitution method. Let's choose , which means .
- Substitution Method: Now we differentiate with respect to to find :
- Differentiate : Substitute with and with in the integral:
- Substitute and : We need to change the limits of integration because we changed the variable of integration from to . When , . When , . So the new limits of integration are from to .
- Change Limits of Integration: Now we can integrate with respect to :
- Integrate : Evaluate the antiderivative at the upper and lower limits:
- Evaluate Antiderivative: Simplify the expression:(\sqrt{2}/2)^4)/4 = (2^2/2^4)/4 = 1/8\(\newline\$(0^4)/4 = 0\(\newline\)\)So the result is \(1/8 - 0 = 1/8\).
- Simplify Expression: The final answer is the value of the definite integral: \(\int_{0}^{\frac{\pi}{4}}\sin^{3}(x)\cos(x)dx = \frac{1}{8}\)