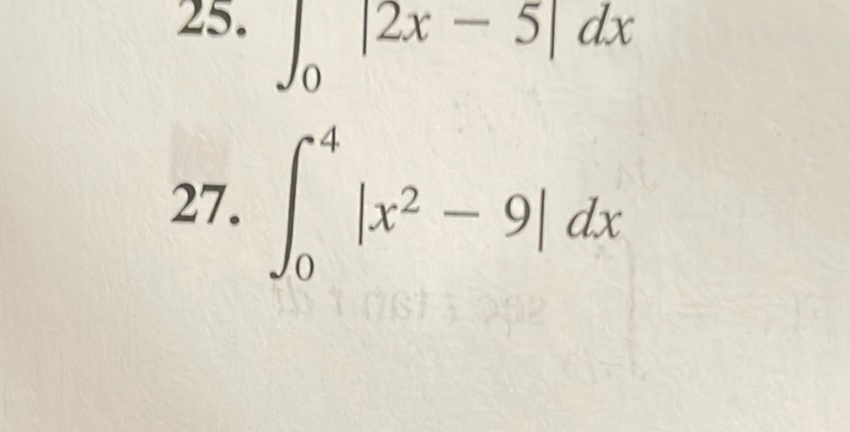AI tutor
Full solution
Q. .
- Identify sign change points: We need to evaluate the integral of the absolute value function from to . To do this, we first need to find the points where the function inside the absolute value, , changes sign, as this will affect the integral.
- Split integral into intervals: The function equals zero when , which happens at and . Since we are only interested in the interval from to , we only need to consider . This is the point where the function changes from negative to positive.
- Evaluate first integral: We split the integral into two parts, from to and from to , and remove the absolute value by considering the sign of the function in each interval. For to , is negative, so we take the negative of the function. For to , is positive, so we take the function as it is.
- Evaluate second integral: Now we write the integral as the sum of two integrals: .
- Add results: We evaluate the first integral: from to .
- Add results: We evaluate the first integral: from to .Plugging in the limits for the first integral, we get .
- Add results: We evaluate the first integral: from to . Plugging in the limits for the first integral, we get . We evaluate the second integral: from to .
- Add results: We evaluate the first integral: from to . Plugging in the limits for the first integral, we get . We evaluate the second integral: from to . Plugging in the limits for the second integral, we get .
- Add results: We evaluate the first integral: from to .Plugging in the limits for the first integral, we get .We evaluate the second integral: from to .Plugging in the limits for the second integral, we get .Now we add the results of the two integrals: