AI tutor
Full solution
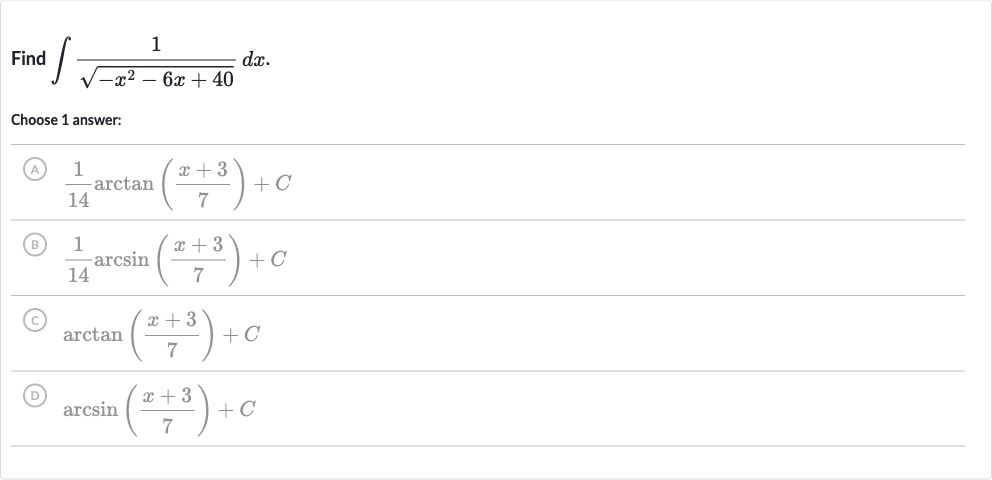
Q. Find .Choose answer:(A) (B) (c) ()
- Recognize inverse trigonometric function: Next, we recognize that the integral is in the form of an inverse trigonometric function, specifically . The general form is . Here, and . So the integral becomes .
- Substitute values: Finally, we check the answer choices to see which one matches our result.The correct answer is (B) .Wait, we made a mistake in the coefficient. It should be not .