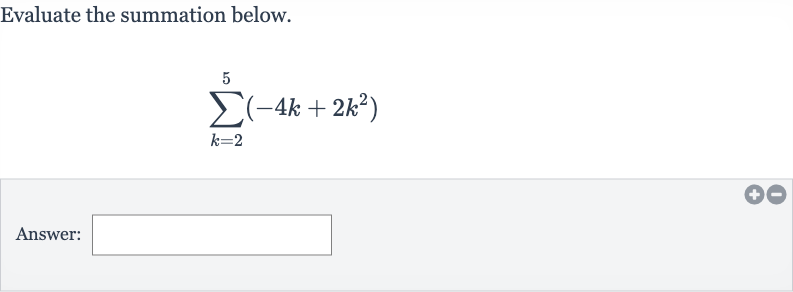AI tutor
Full solution
Q. Evaluate the summation below.Answer:
- Set up the problem: Understand the summation expression and set up the problem.We need to evaluate the sum of the expression for each integer value of from to .
- Calculate for : Calculate the sum for .Substitute into the expression to get the first term of the summation.First term = .
- Calculate for : Calculate the sum for .Substitute into the expression to get the second term of the summation.Second term = .
- Calculate for : Calculate the sum for .Substitute into the expression to get the third term of the summation.Third term = .
- Calculate for : Calculate the sum for . Substitute into the expression to get the fourth term of the summation. Fourth term = .
- Find total sum: Add all the terms together to find the total sum.Total sum = First term + Second term + Third term + Fourth term = .