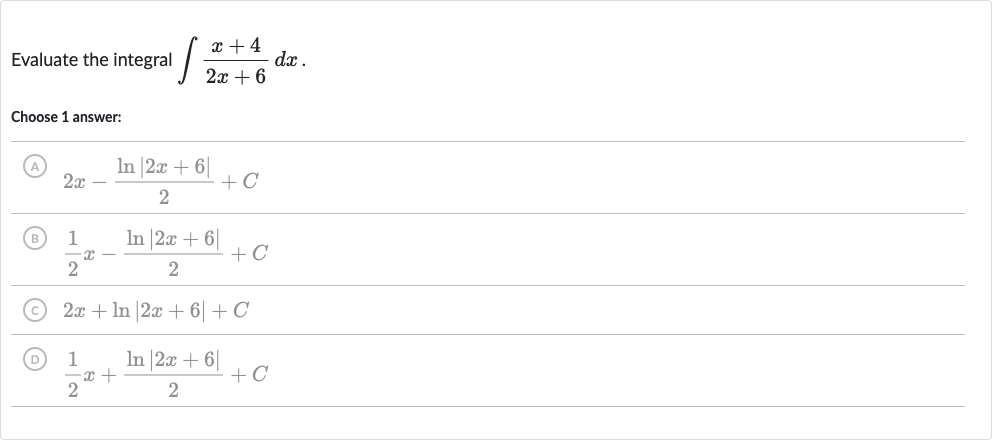
Full solution
Q. Evaluate the integral .Choose answer:(A) (B) (C) (D)
- Partial Fraction Decomposition: Let's do a partial fraction decomposition first. We can write as , where and are constants we need to find.
- Finding Constants and : To find and , we set . Let's choose to make the term disappear and solve for .
- Solving for : Plugging into the equation, we get , which simplifies to .
- Finding B: Now let's find . We can choose to make the term disappear. Plugging into the equation, we get , which simplifies to .
- Final Integration: We now have the partial fraction decomposition: . Let's integrate both terms separately.
- Integral of : The integral of is , because the derivative of is , and we need to multiply by to compensate for the extra .
- Integral of : The integral of is , because the integral of a constant is just the constant times .
- Combining Integrals: Adding both integrals together, we get .