AI tutor
Full solution
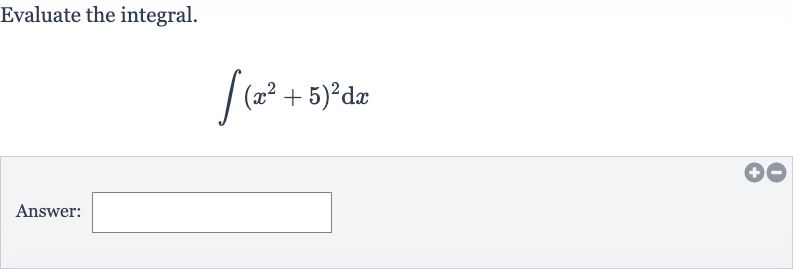
Q. Evaluate the integral.Answer:
- Expand the integrand: We need to expand the integrand before integrating.
- Integrate the expanded form: Now we integrate the expanded form term by term.
- Integrate each term separately: Integrate each term separately using the power rule for integration, which states that for any real number .
- Combine the integration results: Combine the results of the integrations to get the final indefinite integral.