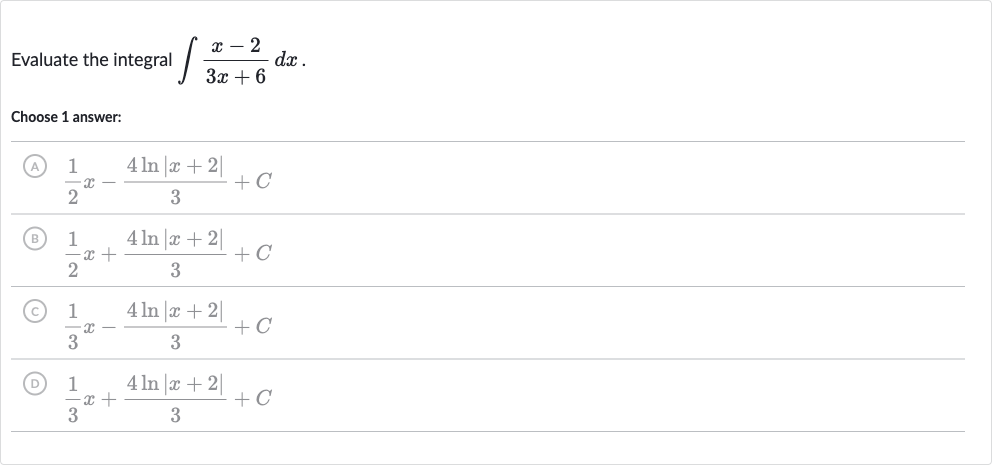
Full solution
Q. Evaluate the integral .Choose answer:(A) (B) (C) (D)
- Rewrite Integral: Rewrite the integral by factoring out the constant from the denominator. \int\frac{x\(-2\)}{\(3\)x+\(6\)}\,dx = \int\frac{x\(-2\)}{\(3\)(x+\(2\))}\,dx
- Split into Two: Split the integral into two separate integrals. \(\int\frac{x-2}{3(x+2)}dx = \frac{1}{3}\int\frac{x}{x+2}dx - \frac{1}{3}\int\frac{2}{x+2}dx
- Simplify First Integral: Simplify the first integral by dividing by .
- Separate Integrals: Separate the integrals.(\frac{\(1\)}{\(3\)})\int(\(1 - \frac{}{x+})dx = (\frac{}{})\int()dx - (\frac{}{})\int(\frac{}{x+})dx
- Integrate Both Terms: Integrate both terms.(\frac{\(1\)}{\(3\)})\int(\(1)dx - (\frac{}{})\int(\frac{}{(x+)})dx = (\frac{}{})x - (\frac{}{})\ln|x+| + C
- Multiply by Constants: Multiply through by the constants.(\frac{\(1\)}{\(3\)})x - (\frac{\(2\)}{\(3\)})\ln|x+\(2| + C = (\frac{}{})x - (\frac{}{})\ln|x+| + C
- Match with Options: Match the result with the given options.The correct answer is (D)