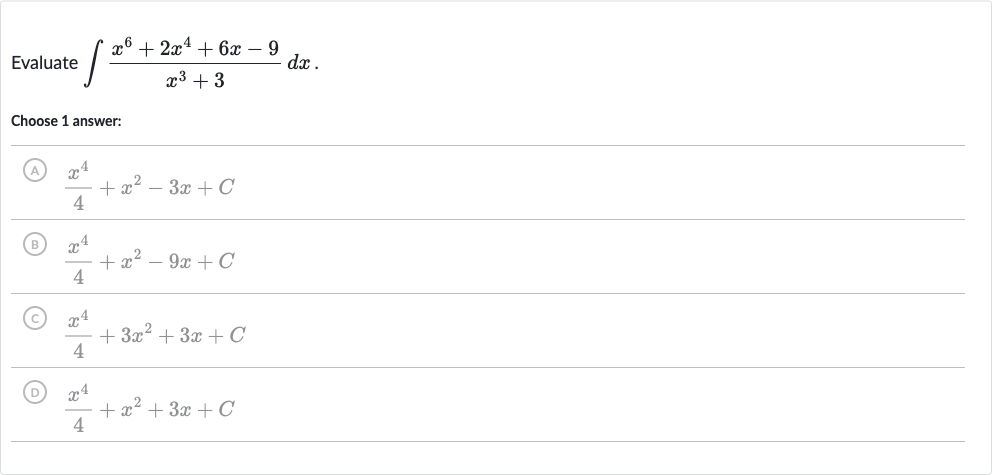AI tutor
Full solution
Q. Evaluate Choose answer:(A) (B) (C) (D)
- Divide and Sum Integrals: Divide by to get , and by to get . The integral becomes the sum of the integrals of , , rac{6x}{x^3+3}, and - rac{9}{x^3+3}.
- Integrate : Integrate to get .
- Integrate : Integrate to get .
- Integrate : Integrate . This is a bit tricky, but notice that the derivative of is , which is close to . Let's use substitution: let , then . We need to adjust for the , so we multiply by to get the . The integral of becomes times the integral of .
- Integrate : Integrate to get
- Substitute back: Substitute back for to get .
- Integrate : Integrate . This is a constant over a linear term, so it's just times the integral of . This doesn't have an elementary antiderivative, so it looks like I made a mistake here. The integral of should be treated as a separate term.