Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Evaluate
int_(6)^(12)(x-7)/(x-5)dx. Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).
Submit Answer

Evaluate $\int_{6}^{12} \frac{x-7}{x-5} d x$ . Write your answer in simplest form with all logs condensed into a single logarithm (if necessary). $\newline$ Submit Answer

View step-by-step help

View step-by-step help

Evaluate definite integrals using the chain rule

Full solution

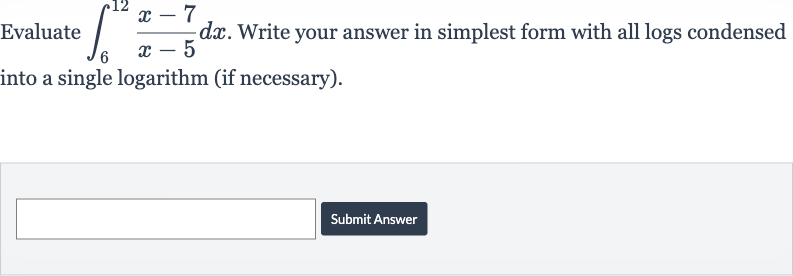
Q. Evaluate

\int_{6}^{12} \frac{x-7}{x-5} d x

. Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).

\newline

Submit Answer

Simplify the integrand: Simplify the integrand. $\newline$ We have the integrand $(x-7)/(x-5)$ . We can perform long division or recognize that this is a proper rational function that can be decomposed into a sum of simpler fractions. We can write $(x-7)/(x-5)$ as $1 - 2/(x-5)$ .
Set up the integral: Set up the integral with the simplified integrand. $\newline$ Now we can write the integral as: $\newline$ $\int_{6}^{12} (1 - \frac{2}{x-5}) \, dx$ $\newline$ This separates into two simpler integrals: $\newline$ $\int_{6}^{12} 1 \, dx - \int_{6}^{12} \frac{2}{x-5} \, dx$
Evaluate first integral: Evaluate the first integral. $\newline$ The integral of $1$ with respect to $x$ from $6$ to $12$ is simply $x$ evaluated from $6$ to $12$ : $\newline$ $\int_{6}^{12} 1 \, dx = [x]_{6}^{12} = 12 - 6 = 6$
Evaluate second integral: Evaluate the second integral. $\newline$ The integral of $\frac{2}{x-5}$ with respect to $x$ from $6$ to $12$ is $2$ times the natural logarithm of the absolute value of $(x-5)$ evaluated from $6$ to $12$ : $\newline$ $\int_{6}^{12} \frac{2}{x-5} \, dx = 2 \times [\ln|x-5|]_{6}^{12}$ $\newline$ $= 2 \times (\ln|12-5| - \ln|6-5|)$ $\newline$ $= 2 \times (\ln|7| - \ln|1|)$ $\newline$ $x$ $0$
Combine results: Combine the results from Step $3$ and Step $4$ . $\newline$ The final answer is the difference between the two results: $\newline$ $6 - 2 \times \ln(7)$
Write final answer: Write the answer in simplest form. $\newline$ Since $\ln(1)$ is $0$ , we can simplify the expression to: $\newline$ $6 - 2 \times \ln(7)$ $\newline$ This is already in simplest form with the logarithm condensed into a single term.

More problems from Evaluate definite integrals using the chain rule

Question

Consider the following problem:

\newline

The water level under a bridge is changing at a rate of

r(t)=40 \sin \left(\frac{\pi t}{6}\right)

centimeters per hour (where

t

is the time in hours). At time

t=3

, the water level is

91

centimeters. By how much does the water level change during the

4^{\text {th }}

\newline

Which expression can we use to solve the problem?

\newline

1

\newline

\int_{3}^{4} r(t) d t

\newline

\int_{0}^{4} r(t) d t

\newline

\int_{4}^{5} r(t) d t

\newline

\int_{4}^{4} r(t) d t

Posted 1 year ago

Question

s(t)

gives the number of students enrolled in a school by time

t

\newline

\int_{15}^{18} s^{\prime}(t) d t=20

\newline

1

\newline

(A) The rate of change of enrollment is

20

students per year more in year

18

than it was in year

15

\newline

20

more students enrolled in year

18

15

\newline

(C) Between years

15

18

, the cumulative number of years of schooling of all of the enrolled students is

20

\newline

20

students enrolled in year

18

Posted 1 year ago

Question

A water tank is filled at a rate of

r(t)

liters per minute (where

t

is the time in minutes).

\newline

\int_{1}^{7} r^{\prime}(t) d t

\newline

1

\newline

(A) The rate at which the tank was filled at

t=7

\newline

B) The average rate of filling between

t=1

t=7

\newline

(C) The change in the rate of filling between

t=1

t=7

\newline

(D) The amount of water filled between

t=1

t=7

Posted 1 year ago

Question

The base of a solid is the region enclosed by the graphs of

y=\sin (x)

y=4-\sqrt{x}

x=2

x=7

\newline

Cross sections of the solid perpendicular to the

x

-axis are rectangles whose height is

2 x

\newline

Which one of the definite integrals gives the volume of the solid?

\newline

1

\newline

\int_{2}^{7}\left[(4-\sqrt{x})^{2}-\sin ^{2}(x)\right] d x

\newline

\int_{2}^{7}[\sin (x)+\sqrt{x}-4] \cdot 2 x d x

\newline

\int_{2}^{7}\left[\sin ^{2}(x)-(4-\sqrt{x})^{2}\right] d x

\newline

\int_{2}^{7}[4-\sqrt{x}-\sin (x)] \cdot 2 x d x

Posted 1 year ago

Question

Evaluate the integral.

\newline

\int-2 x^{3} \cos (3 x) d x

\newline

Posted 1 year ago

Question

Evaluate the integral.

\newline

\int-4 x^{2} 3^{3 x} d x

\newline

Posted 1 year ago

Question

Evaluate the integral.

\newline

\int-4 x^{2} e^{4 x} d x

\newline

Posted 1 year ago

Question

\sum_{n=1}^{\infty}\frac{3^{n}n^{2}}{n!}

Posted 1 year ago

Question

f) \lim_{n \to +\infty}\left[\frac{2}{n}+\left(\frac{3}{5}\right)^n\right]

Posted 1 year ago

Question

\int \frac{1}{\cos(x)^{2}}\,dx

Posted 1 year ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant