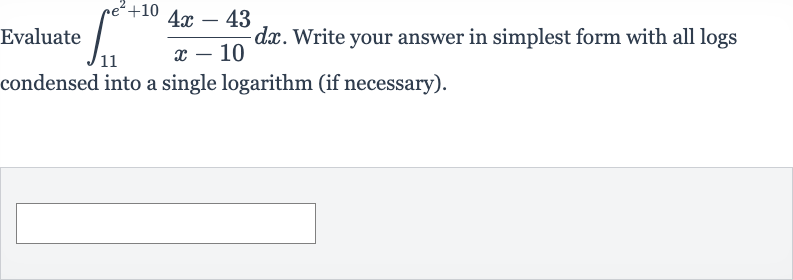
Evaluate int_(11)^(e^(2)+10)(4x-43)/(x-10)dx. Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).

Question

Evaluate  int_(11)^(e^(2)+10)(4x-43)/(x-10)dx. Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).

Bytelearn · Accepted Answer

Simplify Integrand: Simplify the integrand if possible. The integrand (4x-43)/(x-10) can be simplified by long division since the degree of the numerator is equal to the degree of the denominator. We divide 4x by x to get 4 and multiply (x-10) by 4 to get 4x-40. We subtract this from the numerator to get a remainder of -3. So, the integrand simplifies to 4 - 3/(x-10).Split Integral: Split the integral into two separate integrals. The integral of the sum is the sum of the integrals, so we can write: ∫(4x-43)/(x-10) dx = ∫4 dx - ∫3/(x-10) dxIntegrate Terms: Integrate each term separately. The integral of a constant is just the constant times the variable, so: ∫4 dx = 4x The integral of 3/(x-10) is 3 times the natural logarithm of the absolute value of (x-10), so: ∫3/(x-10) dx = 3ln|x-10|Combine Integrals: Combine the two integrals. The combined integral is: ∫(4x-43)/(x-10) dx = 4x - 3ln|x-10|Evaluate Definite Integral: Evaluate the definite integral from 11 to e^(2)+10. We substitute the upper and lower limits into the antiderivative: (4(e^(2)+10) - 3ln|e^(2)+10-10|) - (4(11) - 3ln|11-10|)Simplify Expression: Simplify the expression. We simplify the expression by performing the arithmetic: = (4e^(2)+40 - 3ln|e^(2)|) - (44 - 3ln|1|) Since ln|e^(2)| = 2 (because e^(ln x) = x for any x) and ln|1| = 0 (because e^0 = 1), we get: = (4e^(2)+40 - 3*2) - (44 - 3*0) = (4e^(2)+40 - 6) - (44) = 4e^(2)+34 - 44 = 4e^(2) - 10Write Final Answer: Write the final answer. The final answer is the simplified form of the definite integral from 11 to e^(2)+10 of the function (4x-43)/(x-10).

Evaluate $\int_{11}^{e^{2}+10} \frac{4 x-43}{x-10} d x$ . Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).

Full solution

More problems from Evaluate definite integrals using the chain rule

Related topics

Evaluate ∫11e2+104x−43x−10dx \int_{11}^{e^{2}+10} \frac{4 x-43}{x-10} d x ∫11e2+10​x−104x−43​dx. Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).

Evaluate $\int_{11}^{e^{2}+10} \frac{4 x-43}{x-10} d x$ . Write your answer in simplest form with all logs condensed into a single logarithm (if necessary).