Full solution
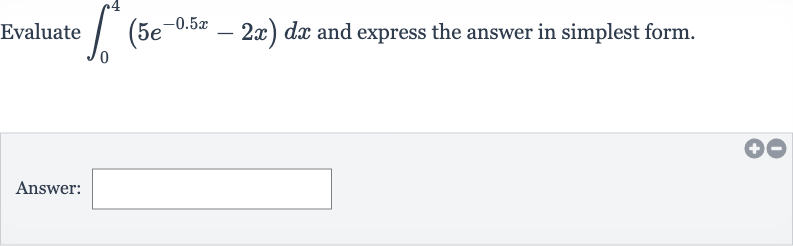
Q. Evaluate and express the answer in simplest form.Answer:
- Substitution Method Integration: We need to evaluate the integral of the function from to . We will integrate each term separately.First, let's integrate . We can use the substitution method for this part of the integral.Let , which implies or .The integral of with respect to is .So, the integral of is .
- Integrating : Next, we integrate with respect to . The integral of with respect to is .So, the integral of is .
- Combining Integrals: Now we combine the two integrals to get the integral of the entire function: , where is the constant of integration.
- Evaluating Antiderivative: We evaluate this antiderivative from to : ] evaluated from to . This gives us: ] - [\(-10e^{(.\cdot)} - ^\]).
- Plugging in Values: Now we plug in the values and simplify:[-10e^{-2} - 16\] - \[-10e^{0} - 0\]).\(\newlineWe know that \$e^{0} = 1\), so this simplifies to:\(\newline\)\([\(-10\)/e^{\(2\)} - \(16\)\] - \[-10 - 0\]).
- Final Answer: Simplifying further, we get:\(\newline\)\(-10/e^2 - 16 + 10\).
- Final Answer: Simplifying further, we get:\(\newline\)\(-10/e^2 - 16 + 10\).Finally, we combine like terms to get the final answer:\(\newline\)\(-10/e^2 - 6\).