Full solution
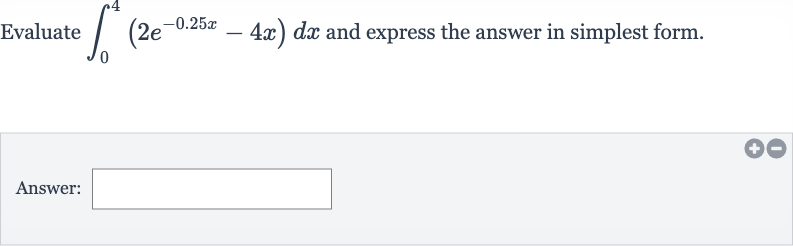
Q. Evaluate and express the answer in simplest form.Answer:
- Break down integral: Break down the integral into two separate integrals.We have the integral of a sum of two functions, which can be separated into the sum of two integrals:\int(\(2e^{.x} - x)\,dx = \int e^{.x}\,dx - \int x\,dx
- Evaluate first integral: Evaluate the first integral . To integrate , we use the substitution method: Let , then , or . The limits of integration also change with the substitution. When , , and when , . The integral becomes: The integral of with respect to is , so we have: Substituting back for , we get:
- Evaluate second integral: Evaluate the second integral . The integral of with respect to is , so we have:
- Combine results: Combine the results of the two integrals.The combined indefinite integral is:
- Evaluate definite integral: Evaluate the definite integral from to . We need to calculate the value of the combined integral at the upper limit () and subtract the value at the lower limit (): Calculating the values, we get:
- Simplify final result: Simplify the final result.Combining the terms, we get: