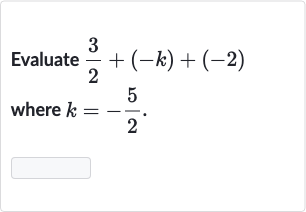AI tutor
Full solution
Q. Evaluate where .
- Substitute value of k: First, let's substitute the value of into the expression. where
- Replace in expression: Now, replace with in the expression.
- Simplify double negative: Simplify the double negative by removing the extra negative sign in front of .
- Combine fractions: Combine the fractions and .
- Simplify fraction: Simplify the fraction .
- Add whole numbers: Now, add the whole number to the negative whole number .
- Perform subtraction: Perform the subtraction.
More problems from Solve advanced linear inequalities
QuestionGet tutor help