Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

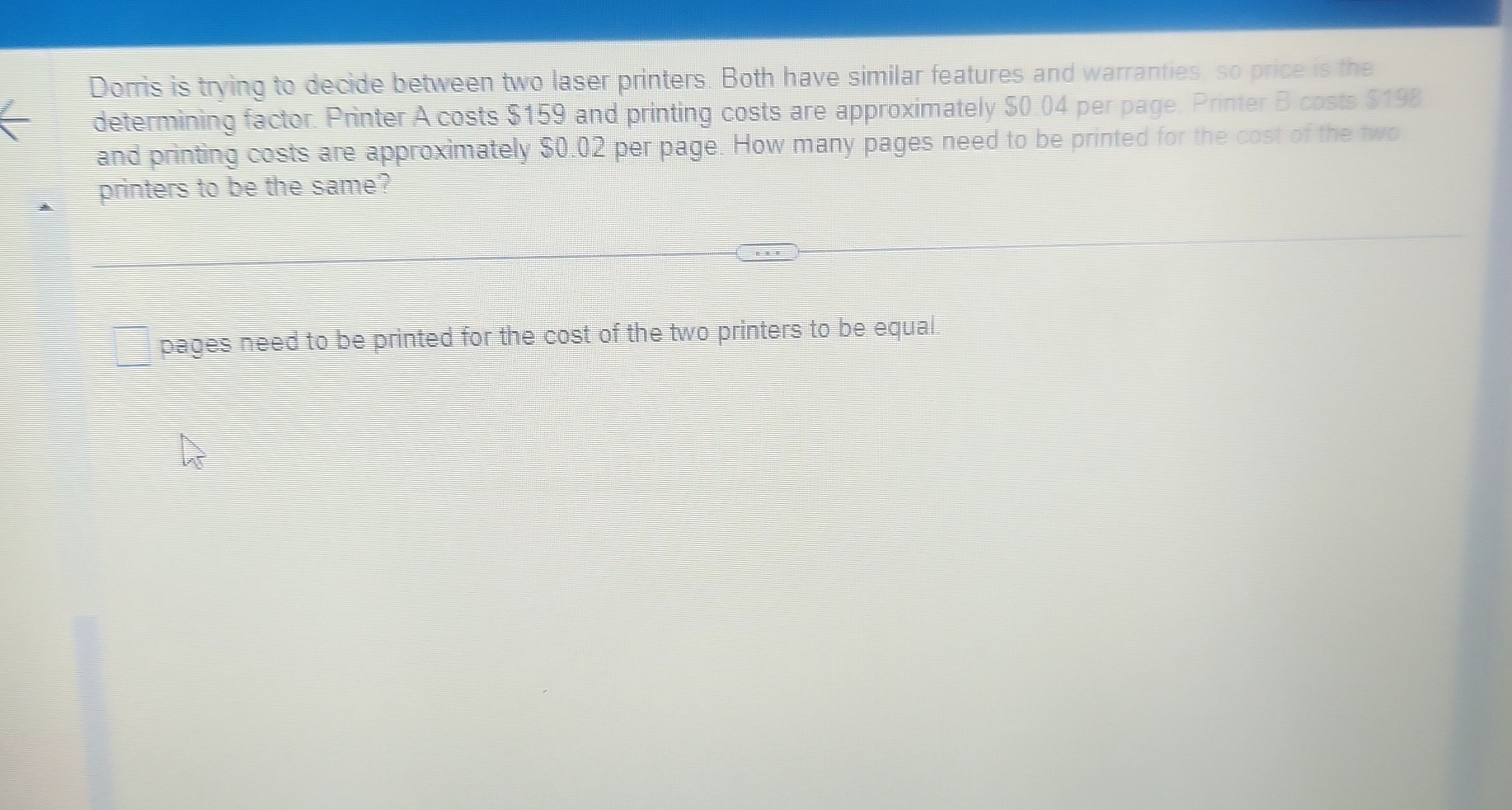
Dorris is trying to decide between two laser printers. Both have similar features and warranties so price is the determining factor. Printer A costs $159 and printing costs are approximately $0.04 per page Printer B costs $194. and printing costs are approximately $0.02 per page. How many pages need to be printed for the cost of the two printers to be the same? pages need to be printed for the cost of the two printers to be equal.

Dorris is trying to decide between two laser printers. Both have similar features and warranties so price is the determining factor. Printer A costs $159 \$ 159 and printing costs are approximately $0.04 \$ 0.04 per page Printer B costs $194 \$ 194 . and printing costs are approximately $0.02 \$ 0.02 per page. How many pages need to be printed for the cost of the two printers to be the same?\newlinepages need to be printed for the cost of the two printers to be equal.

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Solve system of equations: Complex word problemsright chevron icon

Full solution

Q. Dorris is trying to decide between two laser printers. Both have similar features and warranties so price is the determining factor. Printer A costs $159 \$ 159 and printing costs are approximately $0.04 \$ 0.04 per page Printer B costs $194 \$ 194 . and printing costs are approximately $0.02 \$ 0.02 per page. How many pages need to be printed for the cost of the two printers to be the same?\newlinepages need to be printed for the cost of the two printers to be equal.
  1. Identify Cost Difference: Identify the initial cost difference between the two printers. Printer A costs $159\$159 and Printer B costs $194\$194. The difference in initial cost is $194−$159=$35\$194 - \$159 = \$35.
  2. Identify Printing Costs: Identify the difference in printing costs per page between the two printers. Printer A's printing cost is $0.04\$0.04 per page and Printer B's printing cost is $0.02\$0.02 per page. The difference in printing cost per page is $0.04−$0.02=$0.02\$0.04 - \$0.02 = \$0.02.
  3. Set Up Break-Even Equation: Set up the equation to find the break-even point where the total costs of both printers are the same.\newlineLet the number of pages to be printed be represented by 'p'.\newlineThe total cost for Printer A will be the initial cost plus the cost per page times the number of pages: $159+$0.04p\$159 + \$0.04p.\newlineThe total cost for Printer B will be the initial cost plus the cost per page times the number of pages: $194+$0.02p\$194 + \$0.02p.\newlineSo, we have the equation $159+$0.04p=$194+$0.02p\$159 + \$0.04p = \$194 + \$0.02p.
  4. Solve for Number of Pages: Solve the equation for 'p' to find the number of pages.\newline159+0.04p=194+0.02p159 + 0.04p = 194 + 0.02p\newlineSubtract 0.02p0.02p from both sides to get the terms with 'p' on one side:\newline0.04p−0.02p=194−1590.04p - 0.02p = 194 - 159\newline0.02p=350.02p = 35
  5. Divide to Find Value: Divide both sides by $0.02\$0.02 to find the value of 'p'.\newlinep=$35$0.02p = \frac{\$35}{\$0.02}\newlinep=1750p = 1750
  6. Conclude with Final Answer: Conclude with the final answer.\newlineThe number of pages that need to be printed for the cost of Printer A and Printer B to be the same is 17501750 pages.

More problems from Solve system of equations: Complex word problems

Question
Solve using substitution. 5x−2y=−7 5x - 2y = -7 x=−5 x = -5 (_,_)
Get tutor helpright-arrow

Posted 2 months ago

Question
Is (1,1)(1,1) a solution to this system of equations? \newline4x+10y=144x + 10y = 14\newlinex+6y=7x + 6y = 7\newlineChoices:\newline(A) yes \newline(B) no
Get tutor helpright-arrow

Posted 2 months ago

Question
Which describes the system of equations below?\newliney=–3x+9y = –3x + 9 \newliney=–3x+9y = –3x + 9 \newlineChoices:\newline(A) consistent and independent\text{(A) consistent and independent} \newline(B) consistent and dependent\text{(B) consistent and dependent} \newline(C) inconsistent\text{(C) inconsistent}
Get tutor helpright-arrow

Posted 2 months ago

Question
Solve using elimination. \newline7x−8y=−17 7x - 8y = -17 \newline −7x+3y=2 -7x + 3y = 2 \newline (____,____)(\_\_\_\_, \_\_\_\_)
Get tutor helpright-arrow

Posted 2 months ago

Question
Solve. \newlinex=−2 x = -2 \newline−2x+2y=−8 -2x + 2y = -8 \newline(____,____)(\_\_\_\_, \_\_\_\_)
Get tutor helpright-arrow

Posted 2 months ago

Question
Write a system of equations to describe the situation below, solve using any method, and fill in the blanks.\newlineAt a community barbecue, Mrs. Wilkerson and Mr. Hogan are buying dinner for their families. Mrs. Wilkerson purchases 33 hot dog meals and 33 hamburger meals, paying a total of $36\$36. Mr. Hogan buys 11 hot dog meal and 33 hamburger meals, spending $26\$26 in all. How much do the meals cost?\newlineHot dog meals cost $\$_______ each, and hamburger meals cost $\$________ each.
Get tutor helpright-arrow

Posted 2 months ago

Question
Solve the system of equations by substitution.\newline−3x−y−3z=−11-3x - y - 3z = -11\newlinez=5z = 5\newlinex−y+3z=19x - y + 3z = 19\newline(____.____,____)
Get tutor helpright-arrow

Posted 2 months ago

Question
Solve the system of equations by elimination.\newlinex−3y−2z=10 x - 3y - 2z = 10 \newline3x+2y+2z=14 3x + 2y + 2z = 14 \newline2x−3y−2z=16 2x - 3y - 2z = 16 \newline(_,_,_)(\_,\_,\_)
Get tutor helpright-arrow

Posted 1 month ago

Question
Solve the system of equations.\newliney=x2+36x+3 y = x^2 + 36x + 3 \newliney=22x−37 y = 22x - 37 \newlineWrite the coordinates in exact form. Simplify all fractions and radicals.\newline(_,_)(\_,\_)\newline(_,_)(\_,\_)
Get tutor helpright-arrow

Posted 1 month ago

Question
Solve the system of equations.\newliney=−x−24 y = -x - 24 \newlinex2+y2=488 x^2 + y^2 = 488 \newlineWrite the coordinates in exact form. Simplify all fractions and radicals.\newline(_,_) (\_,\_) \newline(_,_) (\_,\_)
Get tutor helpright-arrow

Posted 2 months ago

View all (24)