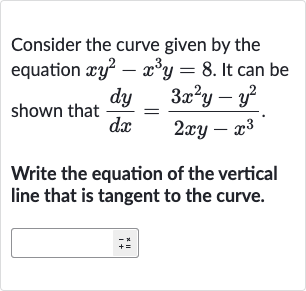
Consider the curve given by the equation xy^(2)-x^(3)y=8. It can be shown that (dy)/(dx)=(3x^(2)y-y^(2))/(2xy-x^(3)). Write the equation of the vertical line that is tangent to the curve.

Question

Consider the curve given by the equation  xy^(2)-x^(3)y=8. It can be shown that  (dy)/(dx)=(3x^(2)y-y^(2))/(2xy-x^(3)). Write the equation of the vertical line that is tangent to the curve.

Bytelearn · Accepted Answer

Set Denominator Equal to Zero: To find the vertical tangent, we need to set the denominator of the derivative (dy/dx) equal to zero, because the slope of a vertical line is undefined, and this occurs when the denominator of the slope expression is zero.Factor and Solve for x: The denominator of the derivative is 2xy - x^3. Set this equal to zero to find the x-values where the tangent line could be vertical. 2xy - x^3 = 0Check x = 0: Factor out x from the equation. x(2y - x^2) = 0Solve for y: Setting each factor equal to zero gives us the x-values where the tangent could be vertical. x = 0 or 2y - x^2 = 0Substitute y into Original Equation: If x = 0, then the equation 2y - x^2 = 0 is automatically satisfied, so we don't get additional information from the second factor. We need to check if x = 0 is indeed a point on the curve.Simplify and Combine Terms: Substitute x = 0 into the original equation xy^2 - x^3y = 8 to see if it yields a valid point on the curve. 0*y^2 - 0^3*y = 8 0 = 8 This is not true, so x = 0 is not a point on the curve, and therefore cannot be where a vertical tangent occurs.Solve for x^5: Now, let's solve the second factor 2y - x^2 = 0 for y to find the corresponding y-values for the vertical tangent. 2y = x^2 y = (1/2)x^2No Real Solution: Substitute y = (1/2)x^2 back into the original equation to find the x-values that satisfy both the original equation and the condition for a vertical tangent. x*(1/2*x^2)^2 - x^3*(1/2*x^2) = 8Simplify the equation. x*(1/4*x^4) - (1/2*x^5) = 8 (1/4*x^5) - (1/2*x^5) = 8Combine like terms. -(1/4*x^5) = 8Multiply both sides by -4 to solve for x^5. x^5 = -32Since x^5 = -32 has no real solution (as the fifth root of a negative number is not real), there are no x-values that satisfy the condition for a vertical tangent on the curve xy^2 - x^3y = 8. Therefore, there is no vertical line that is tangent to the curve.

Consider the curve given by the equation $x y^{2}-x^{3} y=8$ . It can be shown that $\frac{d y}{d x}=\frac{3 x^{2} y-y^{2}}{2 x y-x^{3}}$ . $\newline$ Write the equation of the vertical line that is tangent to the curve.

Full solution

More problems from Find indefinite integrals using the substitution

Related topics

Consider the curve given by the equation xy2−x3y=8 x y^{2}-x^{3} y=8 xy2−x3y=8. It can be shown that dydx=3x2y−y22xy−x3 \frac{d y}{d x}=\frac{3 x^{2} y-y^{2}}{2 x y-x^{3}} dxdy​=2xy−x33x2y−y2​.\newlineWrite the equation of the vertical line that is tangent to the curve.

Consider the curve given by the equation $x y^{2}-x^{3} y=8$ . It can be shown that $\frac{d y}{d x}=\frac{3 x^{2} y-y^{2}}{2 x y-x^{3}}$ . $\newline$ Write the equation of the vertical line that is tangent to the curve.