Full solution
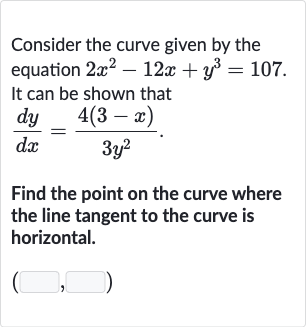
Q. Consider the curve given by the equation . It can be shown that .Find the point on the curve where the line tangent to the curve is horizontal. ,
- Solve for x: Solve for x:
- Find y-coordinate: Now that we have the x-coordinate, we need to find the corresponding y-coordinate on the curve. We plug into the original equation of the curve to find .
- Calculate : Calculate the value of :
- Find : Find the cube root of to get :