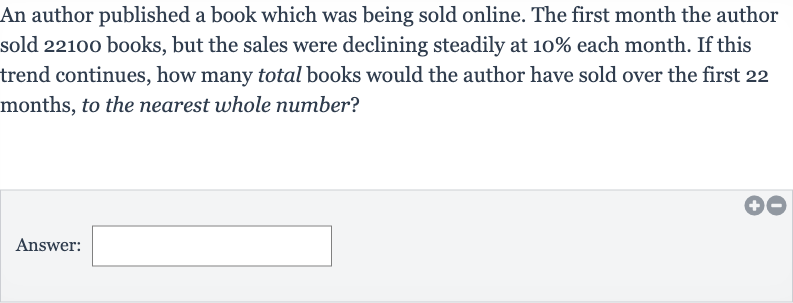
An author published a book which was being sold online. The first month the author sold 22100 books, but the sales were declining steadily at 10% each month. If this trend continues, how many total books would the author have sold over the first 22 months, to the nearest whole number? Answer:

Question

An author published a book which was being sold online. The first month the author sold 22100 books, but the sales were declining steadily at  10% each month. If this trend continues, how many total books would the author have sold over the first 22 months, to the nearest whole number? Answer:

Bytelearn · Accepted Answer

Identify initial number and decrease: Identify the initial number of books sold and the monthly percentage decrease. The author sold 22100 books in the first month and sales are declining by 10% each month.Recognize geometric sequence: Recognize that the situation describes a geometric sequence where each term is 90% (or 0.9 times) of the previous term, because of the 10% decrease. The first term (a1) is 22100, and the common ratio (r) is 0.9.Use formula for sum: Use the formula for the sum of the first n terms of a geometric sequence to find the total number of books sold over the first 22 months. The formula is S_n = a1 * (1 - r^n) / (1 - r), where S_n is the sum of the first n terms, a1 is the first term, r is the common ratio, and n is the number of terms.Calculate sum of first 22 terms: Calculate the sum of the first 22 terms using the formula. S_22 = 22100 * (1 - 0.9^22) / (1 - 0.9)Perform calculations: Perform the calculations. S_22 = 22100 * (1 - 0.9^22) / 0.1 First, calculate 0.9^22. 0.9^22 ≈ 0.095617924991195Calculate 0.9^22: Continue the calculation by subtracting this value from 1. 1 - 0.095617924991195 ≈ 0.904382075008805Subtract from 1: Divide this result by 0.1 to find the multiplier for the initial number of books sold. 0.904382075008805 / 0.1 ≈ 9.04382075008805Divide by 0.1: Multiply this value by the initial number of books sold to find the total number of books sold over the first 22 months. 22100 * 9.04382075008805 ≈ 199969.45Multiply by initial number: Round the result to the nearest whole number, as the question asks for the total number of books to the nearest whole number. 199969.45 rounds to 199969.

Full solution

More problems from Percent of change: word problems

Related topics